Waves A-level revision questions with answers

1. (a) What is meant by interference pattern as applied to waves. (03marks)

When two sound waves meet they superpose. The interference pattern produced depends on the path difference.

If the path difference is a whole number, multiples of wavelength of wave, reinforcement takes place resulting into bright fringes. i.e. path difference, ∆ = nλ, n = 0, 1, 2, …..

If the path difference in an odd number, multiple of half-wavelength, cancellation takes place resulting into regions of dark fringe; i.e. path difference,  

If the sources are coherent, permanent alternative regions of bright and dark fringes are formed and are called interference pattern. Thus interference has occurred.

(b) Explain why it is necessary to use a common source when demonstrating interference in light (03marks)

  To produce observable fringes,

• The waves should have constant phase difference between them.
• The amplitude of the waves should also be equal or nearly equal

Since emission of light is always spontaneous; the above conditions are only possible if the source is the same.

(c) In an experiment to determine wavelength of light using Young’s method, two slits, a separation of 1.2mm were used. When the screen was placed 18.0cm from slits, 30 bright fringes occupying a distance 2.5mm was obtained,

(i) Find wavelength of light used (04marks)

Slit separation, a= 1.2 x 10^-3m

            Number of bright fringes, n =30

            Distance from center of nth fringe, x_n = 2.5 x 10^-3m

            Distance from the slits to screen, D = 18.0cm = 0.18m

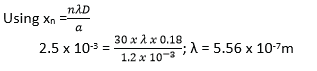
(ii) List the changes that would be observed if the distance of the screen from the slits was increased (02marks)

  If the distance, D, of the screen from slits is increased, it follows from   that the fringe              separation, y, also increases, keeping wavelength, λ and slit separation, a constant.

      Hence fringes occupy a bigger area on screen but their brightness reduce (become less distinct).

(d)(i) Derive the expression for angular position of n^th order principal maximum produced by transmission diffraction grating.

 Solution

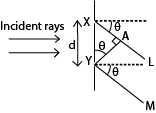
Suppose X and Y are corresponding points in consecutive slits where XY = d, and the grating is illuminated normally by monochromatic light of wavelength, λ, the direction, θ, the diffracted ray XL, and YM have path difference, XA

The path difference for all corresponding points in the two slits have the same path difference, dsinθ. So other pairs of slits throughout the grating are treated the same way.

Also for bright or principle maxima to be obtained, the path difference = nλ.

  Thus,   dsin θ = nλ; where d= slit separation, λ = wavelength, θ = angular momentum

(ii) Light of two wavelength 5.4 x 10^-7m and 5.7 x 10^-7m incident normally on transmission grating with spacing 2.00 x 20^-6m. Find the angular separation of second order principal maxima.

(iii) Suppose white light is used in (d)(ii) , describe the positions of the red and violet lights in the order principal maximum relative central maxima (01mark)

Violet will nearer to central maximum while red will be farther off.

 

2. (a) Define the following

(i) Transverse waves

It is a wave in which the wave particles vibrate perpendicular to the direction of the wave motion

(ii) Longitudinal waves

It is a wave in which the wave particles vibrate parallel to the direction of the wave motion.

(b) When a plane wave transverse a medium, the displacement of the particle is given by

y = 0.01sin2π(2t-0.01x), where y and x are in meters and t in seconds

Calculate the

(i) Frequency of the wave (02marks)

Form y = Asin2π(ωt-kx)

A= 0.01m, ω = 2π(2), x = 2π(0.01)

Frequency, f, of a wave is given by ω = 2πf

4π = 2πf

f = 2Hz

(ii)Wave velocity (03marks)

(iii) Phase difference at a given instant of time between two particles 50m apart. (02marks)

(c) Describe an experiment to determine the velocity of sound in air by an interference method. (06marks)

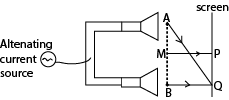
Two small loud speakers A and B about 1m apart are connected to the same oscillator so that both emit sound waves of frequency, f, in phase.

A sensitive detector is moved parallel to the line AB along PQ and it detects a maximum wave at P on perpendicular bisector MP of AB and another maximum wave when it first reaches a point Q directly opposite to B.

Constructive interference of sound wave occurs at P and Q, so the wavelength of sound wave is given by λ = AQ –BQ

The speed of sound in air is then calculated from V =fλ.

Alternative 1

A long tube is filled with water.

A vibrating turning fork of known frequency f is held over the mouth of the tube.

Water is gradually removed until a loud sound is heard.

The length l₁ of air column is measured.

Water is allowed out until a loud sound is heard again.

The length l2 of air column is measured

The velocity of sound in air is calculated from V= 2f(l₂ –l₁)

Alternative 2

(i) Along tube is filled with water.

(ii) A vibrating turning fork of known frequency f is held over the mouth of the tube.

(iii) Water is gradually removed until a loud sound is heard.

(iv) The length L of air column is measured.

(v) (i) to (iv) is repeated with five other different turning forks

(vi) The results are tabulated including values of

(vii) A graph of L against is plotted and slope S is determined.

(viii) The speed of sound, v = 4S.

(d) (i) state two applications of Doppler effect. (02marks)

• Estimation of speed of distant objects like star and planets
• To detect objects moving in a circular track, for example a car negotiating a corner towards or away from an observer.
• Used in some types of radars to measure the velocity of detected objects, for example a motor car. The police use radar to detect speeding motorist as it approaches or recedes from a radar source
• In measurement of plasma temperature.

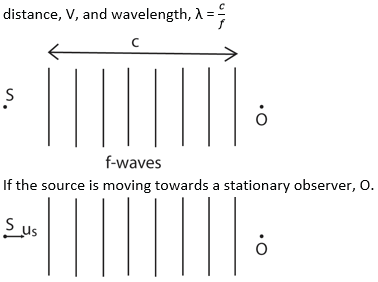
(ii) Derive an expression for frequency, f, of sound as heard by stationary observer when the source of sound of frequency, f, approaches with uniform speed, u_s (03marks)

Suppose c is the speed of sound in air, u_s is the speed of source, S, of sound, f is the frequency of source

If the source S were stationery, the f-wave sent out in 1s towards observer would cover a distance,

 

3. (a) define the following as applied to wave motion

(i) Amplitude (01mark)

This is the maximum displacement of a wave particle from its equilibrium position.

(ii) Frequency (01mark)

This is the number of complete cycles per second.

(iii) Wavelength (01mark)

This is the distance between two successive wave particles that are in phase or the distance between two successive troughs or crests.

(vi) Derive the relationship between velocity, wavelength and frequency of a wave (03marks)

(c) (i) What is meant by the Doppler effect? (01marks)

It is the apparent change in frequency of a wave motion due to relative motion between the source and observer.

(ii) A source of sound moving with velocity, u. approaches an observer moving with velocity u₀ in the same direction. Derive the expression for frequency of sound heard by observer. (05marks)

(d) Two whistles are sounded simultaneously. The wavelengths of the sounds emitted are 5.5m and 6.0m respectively. Find the beat frequency if the speed of sound is 330ms^-1.

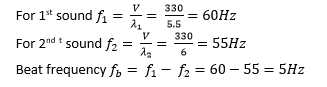
1. 4. (a) What is meant by the following as applied to light waves

(i) Diffraction (01mark)

It is the spreading of light around an obstacle in geometrical shadow leading to interference

(ii) Polarization                 (01mark)

It is a process by which vibration of light (electric vector) photons are restricted in one plane perpendicular to the direction of propagation of light.

(b) A diffraction grating of spacing d, is illuminated normally with light of wavelength, λ.

(i) Derive the condition for concurrence of diffraction maxima. (03marks)

Consider a parallel beam of monochromatic light incident on a grating of spacing d.

The path difference XA = dsinθ

For constructive interference, diffraction maxima occurs when the path difference is an integral multiple of wavelength, λ.

i.e. path difference = nλ

thus dsinθ = nλ where n = 0, 1, 2, 3, ……….

(ii) Describe briefly the intensity distribution on screen placed beyond the grating (02marks)

The central principle maxima is the most intense; the intensity decreases on the either side.

(iii) What is the effect on diffraction pattern when grating with larger number of lines is used (02marks)

The diffraction maxima (image) becomes narrower and shaper

(c) Light of wavelength 5.8 x 10^-7m is incident on diffraction grating of 500 lines per mm. Find the

(i) Diffraction angle for second order image (03marks)

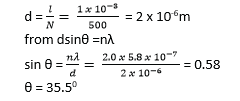
(ii)Maximum number of images formed. (02marks)

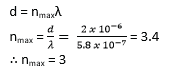
(d) (i) Describe how polarized light can be produced by reflection.        (04marks)

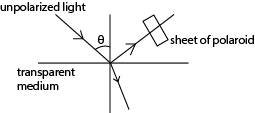
• Unpolarized light is made incident on a transparent medium at an angle θ.
• The reflected light is viewed through a sheet of polaroid while turning the sheet about an axis perpendicular to its plane
• The procedure is repeated for various angles of incidence until the reflected ray is cut off from object.
• At this particular angle θ, the reflected light is completely polarized.

(ii) List for used of polarized light.

• Measurement of sugar concentration
• Determination of stress distribution in materials like glass
• In film to give illusion of three dimension (orography)
• In liquid crystal display

5. (a) Define the following as applied to a wave

(i) Amplitude

This is the maximum displacement of a wave particle from its equilibrium position.

(ii) Wavelength (01mark)

This is the distance between two successive wave particles that are in phase or the distance between two successive troughs or crests.

(b)(i) State the conditions for the formation of standing wave (02marks)

• Two waves must be travelling in opposite direction.
• The waves must have the same speed, frequency and approximately the same amplitude

(ii) A string fixed at both ends is made to vibrate in different modes. If the frequencies of n^th harmonic and fundamental note are f_n and f_l respectively. Show that f_n =nf_l.

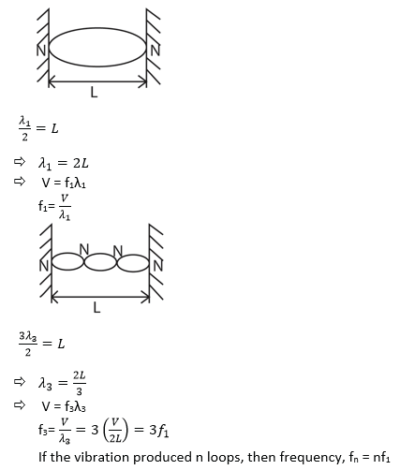
(c) The mass of a vibrating length of sonometer wire is 1.20g. a note of frequency512Hz is produced when the wire is sounding in second over note. If the tension in the wire is 100N. Calculate the vibrating length of the wire. (04marks)

(d) Explain why the quality of a note from an open pipe is preferred to that given by closed pipe (03marks)

Closed pipes give only odd harmonic while open pipes give both odd and even harmonic tones. The more overtone the better the quality of sound.

(e) Describe an experiment to investigate the variation of frequency with length for vibrating wires (05marks)

A sonometer below is used

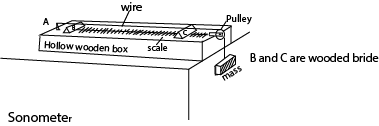
• The wooden bridges B and C vary the effective length of the wire, L.
• Constant tension in the wire is maintained by the fixed mass
• A paper rider is place on the wire in the middle of BC and a sounding fork placed near it.
• The position of the bridge C is varied until sound is heard.
• The distance between the bridges L and the frequency, f, of the tuning fork is noted.
• The procedure is repeated for various tuning forks and values of L, f and 1/L are tabulated
• A plot of f against 1/L gives a straight line showing that the frequency of vibration of the wire is inversely proportional to length.

 

6. (a) Define an optical path (01mark)

This is the product of the refractive index of a medium and the geometrical path length.

Or

It a length of a vacuum that contains the same number of waves as a given length in a medium.

(b) With reference to Young’s double slit experiment,

(i) Explain how interference pattern is formed.

• When two coherent waves travel in a medium, they meet and superimpose.
• Where they meet in phase, constructive interference takes place and intensity is increased (or maximum)
• Where they meet out of phase, cancellation takes place and intensity reduces (minimum)
• Patterns of alternative regions of maximum and minimum intensities are formed that form interference pattern.

(ii) State what happens to the fringes when the source of light is moved nearer to the slits.

  The intensity of fringes increase

(iii) state what happens to fringes when the separation of slits is changes

Increase in separation of slits reduces fringe separation.

(iv) describe the appearance of fringes when white light is used

When white light is used, colored fringes are observed. The central fringe is white, followed by blue and red fringes further off. Outwards the color overlap leading to white illumination

v) Calculate the separation of the slits if the distance from slits to the screen is 800mm and the 8^th         bright fringe is formed 5mm from the center of the fringe system given that the wavelength of           light is 6.2 x 10^-7m

 

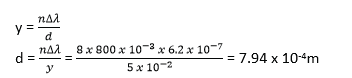
(c) An air wedge is formed by placing two glass slides of length 5.0cm in contact at one end and              separated by a wire on the other end as shown below

When the slides are illuminated with light of wavelength 500nm, 10 dark fringes are observed            to   occupy a distance of 2.5mm

(i) Explain how the fringes are formed (03marks)

• Light is partly reflected and partly transmitted at the lower surface of the top slide.
• The transmitted light is partly reflected on the top of the lower slide.
• The two waves meet and superpose.
• Where the geometrical path difference is an integral multiple of a full wavelength, a dark fringe (band) is formed.
• Where the geometrical path difference is an odd multiple of half wavelength, a bright band is formed. This is because the wave reflected at the surface of the lower slide suffers a phase change of π (180⁰) equivalent to extra path of λ/2  .

(ii) Determine the diameter of the wire(04marks)

 

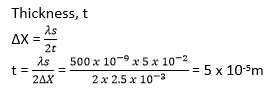
7. (a) What is meant by the following as applied to waves

(i) Resonance

Resonance is said to occur when a body is vibrate at natural frequency due to impulses received from nearby source of the same frequency.

(ii) Frequency

Number of complete cycles made per second.

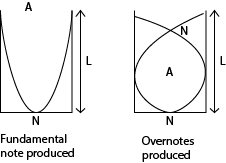
(b) Explain with the aid of a diagram the term fundamental note and overtone as applied to vibrating air in closed pipe.

When the air is brown into a pipe, it vibrates in many different modes, producing waves of different frequencies.

The note of lowest frequency is dominant one and is said to be the fundamental note.

A fundamental note is formed by a wave of large amplitude and with a node N at the closed end and an antinode A at the open end.

The other notes have frequencies higher than that of the fundamental note and are usually have low amplitudes and are less intense. They are called over notes.

 

(c) Describe how you would determine the speed of sound in air using a resonance tube and several tuning forks.

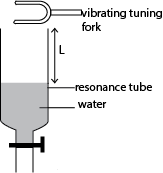
• A glass tube which can be drained from bottom is filled with water.
• A sounding tuning fork of frequency f is brought to the mouth of the tube.
• The water is slowly drained until a loud sound is heard. The tap is closed and length L₁ is measured.
• The tuning fork is again sounded  at the mouth of the tube and the water drained further until a loud sound is heard and distance L₂ is noted
• Velocity, v, of sound in air = 2f(L₂-L₁)

 

(d) (i) Explain the formation of beat.

When two notes of slightly different frequencies but similar amplitudes are sounded together, they superpose producing a note whose intensity increases and reduces periodically. This phenomenon is called beats.

(ii) Derive expression for beat frequency.

Consider two notes of frequencies f₁ and f₂ sounded together. Suppose the wave of frequency f₁ makes one cycle more than that of f₂ in time T₀.

In time T, the number of waves of f₁ is f₁T

The number of waves of f₂ is f₂T

Therefore f₁T – f₂T = 1

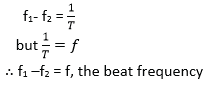
(b) Two observers A and B are provided with sources of sound of frequency 750Hz. If A remains stationary while B moves away at a velocity of 2.0ms^-1, find the number of beats heard per second by A. (Velocity of sound in air is 330ms^-1)

Solution

A hears a note of frequency 750Hz due to its own source. He also hears a note of frequency, f’ due to B.

 

8. (a) What is meant by diffraction?

This is the spreading of light waves beyond their geometrical boundaries leading to interference.

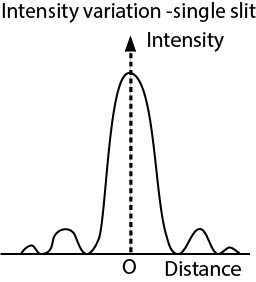
(b) Explain using Huygens’s principle the diffraction pattern produced by a single slit.

• The diffraction patterns formed are due to superposition of secondary wavelets formed from the main wave front at AB
• Each point on the wave front in the gap AB acts as a secondary source of wavelets that propagate in forward direction
• At O, most of the wavelets arrive in phase causing constrictive interference leading to formation of the central bright band.
• On outwards from O, an increasing number of wavelets arrive out of phase and hence the bright bands reduces in intensity until a dark band is formed where all wavelets arrive out of phase.
• As we move farther away from O parallel to AB, the intensity rises again to a much smaller maximum producing less bright band than at O.
• More and more alternative bright and dark bands of reducing intensities are formed further from O parallel to AB where wave wavelets meet in phase and out of phase respectively.

The graph of intensity against distance is shown below.

(c)  Light of wavelength 5.0 x 10^-7m falls on a grating with 600 lines per mm, determine the                        highest  order of diffraction that can be observed.

(d) (i) Explain what is meant by plane of polarized light?

A plane polarization of light is on in which the electric vector of polarized light varies or vibrates.

Plane polarized light is one whose electrical vectors varies in only one plane perpendicular to the direction of travel of the wave.

(ii) One application of polarized light.

Determination of concentration of glucose

(e) A liquid of refractive index 1.3 is used to produce polarized light by reflection. Calculate the                 angle of incidence of incidence on the liquid surface.

(i) Describe how polarized light can be produced by reflection

Solution

• A narrow beam of light is made incident on transparent medium.
• The reflected light is observed through a Polaroid.
• The angle of incidence is varied
• At each angle of incidence, the polaroid is rotated about the axis along the light incident on it
• At one angle of incidence, the light gets cut off the observer as the Polaroid is rotated.
• The reflected ray is now completely polarized.

(ii) State two uses of polarized light.

• Measurement of sugar concentration
• Determination of stress distribution in materials like glass
• In film to give illusion of three dimension (orography)
• In liquid crystal display

 

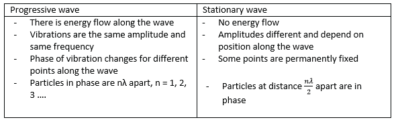
9. (a) Distinguish between progressive and stationary waves

Progressive waves	Stationary waves
(i) There is energy flow along the wave (ii)  Vibrations  have the same amplitudes and frequency (iii) Phases of vibrations changes for different points along the wave (iv) Particles in phase are nλ apart where n = 1,2,3 …….	(i)   No energy flow (ii)  Amplitudes are different and depend on position along the wave (iii)Some points are permanently fixed (iv)  Particles at distance  apart 3nλ/2 are in phase

(b)(i) What are overtones?

Overtones are notes of higher frequencies produced by an instrument after fundamental (or main) notes.

(ii) Explain why a music tone played on one instrument sound differently from the same note                  played on another instrument.

• When an instrument is played, all the allowed vibration take place producing different frequencies.
• The quality of the musical note is determined by the number and strength (intensity) of the overtones.
• Thus when the note played on an instrument has fewer overtones, it sounds different from the same note played on different instrument with more overtones.

(c) A stretched string of length L, is fixed at both ends and then set to vibrate in its allowed modes.        Derive an expression for frequency of the second overtone in terms of fundamental frequency.

(d) A wire of length 0.60m and mass 9 x 10^-4kg is under tension of 135N. The wire is plucked that it vibrates in its third harmonic. Calculate the frequency of the third harmonic.

(e) Describe the variation of pressure with displacement of air in a closed pipe vibrating with fundamental frequency.

At the mouth, air is free to move and therefore the displacement of air molecules is large and the pressure is low. At the closed end, the molecules are less free and the displacement is minimal (or zero) and the pressure is high.

10. (a) What is meant by the following as applied to waves

(i) Phase difference

It is the difference in phase angles of two waves at a given time. Or it is a difference in phase angles of two points in a wave.

(ii) Optical path difference

It is n₁x₁ – n₂x₂, where n₁ and n₂ are refractive indices of media and x₁ and x₂ are the respective geometrical paths.

Or

It is the difference in the length of paths taken by two light waves from the source to the point of meeting.

Or

It is the difference in length of paths taken by two wave trains from coherent sources in the same time interval.

(b)(i) Explain how interference fringes are formed in an air wedge.

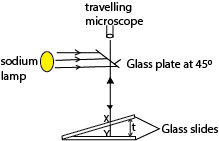
• Light from the lamp is reflected by the inclined glass plate so that it is incident on the wedge normally.
• On reaching X, some of the light is reflected at the bottom part of the top glass slide while part is transmitted and some is reflected at Y on the top part of the bottom glass slide.
• The two reflected waves are coherent and
• The wave reflected at x suffers no phase change while that from Y reflected from at boundary with a denser medium suffers a phase change of π due to reflection which is equivalent to an additional path of 
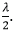
• Therefore a dark band is formed at the point of contact of the slide.
• Where the path difference is an integral multiple of wavelength, a bright band is formed i.e. when

                       where m = 0, 1, 2, 3, … due to reinforcement.

• Where the path difference is an odd multiple of half the λ, a dark band is formed i.e., when    2t = mλ; where m = 0, 1, 2, 3, …

(ii) Two glass slides are separated by a thin wire to form an air wedge. When the wedge is illuminated normally by light of wavelength 5.6 x 10^-7m, a total of 20 fringes occupying a distance of 15mm are obtained. Calculate the angle of the wedge.

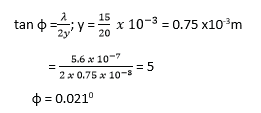
(c) In Young’s double slit experiment, 21 bright fringes occupying a distance of 3.6mm were visible on the screen. The distance of the screen from double slit was 29cm and the wavelength of the light used was 5.5 x 10^-7m.Calculate the separation of the slit.

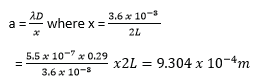
(d)(i) Describe how plane polarized light can be produced by double refraction

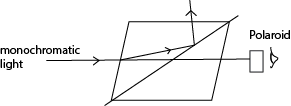
• A narrow beam of monochromatic light is made incident on nicol prism and viewed from the opposite side as shown above
• The angle of incidence is gradually increased or changed and at each angle, the Polaroid is rotated about an axis through its plane.
• At one point, the emergent light is plane polarized.

(ii) Describe practical use of polarized light

• Polarized light is used in measurement of the concentration of sugar in a solution

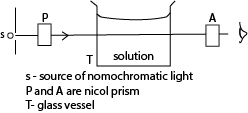
• The apparatus are arranged as above
• First without the solution, the prism A called analyzer is rotated until the emergence light from T is completely extinguished. This orientation is noted.
• T is filled with sugar solution, on looking through A, light can now be seen.
• A is then again rotated until light is extinguished. This point is noted.
• The angle θ between the two positions is noted.
• The angle of rotation θ is proportional to the concentration.

 

• It is used in stress analysis

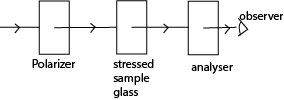
• The apparatus is arranged as above
• The polarizer and analyzer are Polaroid which are crossed with respect to each other so that light does not pass through.
• When a piece of stressed glass is placed between the Polaroids, a pattern of interference fringes can be seen.
• The intensity of light depends on the degree if stress and hence the region and degree of stress is determined.

11. (a) What is meant by the following as applied to wave motion?

(i) Wavelength

       This is the distance between two successive particles in phase

(ii) Wave front

       This is a section through advancing wave on which all particles are in phase.

(b) (i) Define Resonance

        Resonance is a condition obtained when the forcing frequency of an oscillation is equal to the            natural frequency of an oscillating (vibrating) object.

(ii) Describe how velocity of sound can be determined using a resonance tube.

 

• A glass tube which can be drained from bottom is filled with water.
• A sounding tuning fork of frequency f is brought to the mouth of the tube.
• The water is slowly drained until a loud sound is heard. The tap is closed and length L₁ is measured.
• The tuning fork is again sounded  at the mouth of the tube and the water drained further until a loud sound is heard and distance L₂ is noted
• Velocity, v, of sound in air = 2f(L₂-L₁)

(c) (i) Explain how stationary waves are formed.

When two waves of nearly equal frequency and similar amplitude are sounded together, they superpose.

When they meet in phase, constructive interference takes place and loud sound is heard.

When they meet completely out of phase destructive interference takes place and soft sound is heard.

The periodic rise and fall in intensity of sound heard is called beats.

 

(ii) A tuning fork of 760Hz is sounded near the open and closed pipe of length 40 cm. f sir in the tube resonates with the tuning fork, determine the mode vibration and  the end correction. (Velocity of sound in air is 300ms^-1).

Assuming negligible end correction

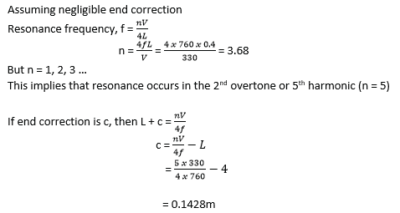
(d)(i) Explain reverberation as applied to sound waves

Reverberation is the prolongation of sound after the sound source has been stopped due to large number of reflected waves which can be perceived by the brain as a continuous sound

(ii) Explain how reverberation can be minimized in a large hall.

Reverberation can be reduced by using materials such as fibre board, heavy curtains, plastics that absorb sound as it reaches the walls and ceiling of the room and t prevent reflection sound

12. (a) (i) state two conditions necessary for interference patterns to be formed.

• The two sources must be coherent; i.e. they must have the same wavelength and be in phase or have constant phase difference.
• The sources must be close to each other.

(ii) With the aid of a diagram, describe how interference can be produced by division of wave                  front.

Solution

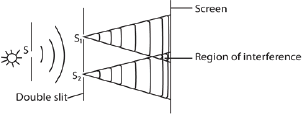
• S, S₁ and S₂ are narrow slits parallel to each other. S diffracts light that falls on it and also illuminates both S₁ and S₂.
• Diffraction also takes place at S₁ and S₂ and interference occurs in the region where light from S₁ overlaps that from S₂.
• Since S is narrow, the light which emerges from S₁ and s₂ comes from the same wave front from S and thus coherent.

 

NB: The coherent sources are obtained by dividing the wave front, originating from a common source, by employing mirrors, biprisms or lenses. This class of interference requires essentially a point source or a narrow slit source.

 

(b) In Young’s double slit experiment, the slits are 0.5mm apart and interference is observed on the screen placed a distance of 100cm from the slits. It is found that the 9^th bright fringe is at a distance of 8.84cm from the second dark fringe from the center of the fringe patterns. Find the wavelength of light used.

(c) Explain what is observed on the interference pattern fringes in Young’s double experiment when the monochromatic source of light is replaced by a source of white light?

A central white fringe with colored edges is observed.

White light consists of several component colours. The interference pattern due to the different component colors of white light overlapping.

At the center of screen, the path difference is zero for all colors. Hence at the center there is a white fringe. Since the violet color has the lowest wavelength, then a violet fringe will be the first fringe on the either side of the central white fringe. The farthest fringe is red

After a few fringes, the fringe pattern become invisible due to much overlapping.

 

(d) Describe how interference fringes are formed in wedge shaped film of air.

 

• Light from the lamp is reflected by the inclined glass plate so that it is incident on the wedge normally.
• On reaching X, some of the light is reflected at the bottom part of the top glass slide while part is transmitted and some is reflected at Y on the top part of the bottom glass slide.
• The two reflected waves are coherent and
• The wave reflected at x suffers no phase change while that from Y reflected from at boundary with a denser medium suffers a phase change of π due to reflection which is equivalent to an additional path of 
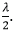
• Therefore a dark band is formed at the point of contact of the slide.
• Where the path difference is an integral multiple of wavelength, a bright band is formed i.e. when

                       where m = 0, 1, 2, 3, … due to reinforcement.

• Where the path difference is an odd multiple of half the λ, a dark band is formed i.e., when    2t = mλ; where m = 0, 1, 2, 3, …

 

13. (a) (i) Distinguish between free oscillation and damped oscillation.

Free oscillation are oscillations in which the total energy of oscillating system is constant (and the amplitude of oscillation is constant) while a damped oscillation is where the energy of the system decreases with time and the amplitude decreases.

(ii) What is meant by resonance as applied to sound?

Resonance is said to occur when a system is set to vibrate at its natural frequency due to impulses received from nearby system vibrating at the same frequency.

(b) Describe an experiment to determine the velocity of sound in air using tuning forks of different frequencies and resonate tube.

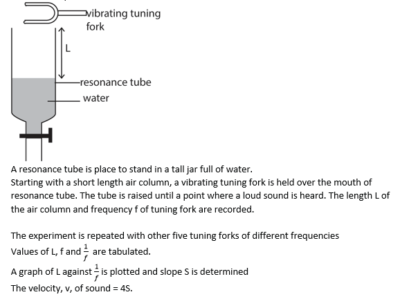
(c) A uniform tube of 80cm long is filled with water and a small loudspeaker connected to signal generator is held over the open end of the tube. With the signal generator set at 600Hz, the water level in the tube is lowered until resonance is first obtained when the length of air column is 13cm. If the third resonance is obtained when the air column is 69.8cm long; calculate the

(i) Velocity of sound

Velocity of sound in air, v = λf = 0.56 x 600 = 340.8ms^-1

(ii) Fundamental frequency for the tube is it were open at both ends.

(d) (i) What is meant by Doppler Effect?

This is the apparent change in the frequency of a wave, when there is relative motion between the source and observer.

(ii) A motor cyclist and police car are approaching each other. The motor cyclist is moving at 10ms^-1 and the police car at 20ms^-1. If the police siren is sounded at 480Hz. Calculate the frequency of the note heard by the cyclist after the police car passes by.

(iii) Give two applications of the Doppler Effect.

• Determining directional motion of stars
• Estimating speed of stars
• Estimating speed of cars using a speed gun
• Determination of plasma temperature.

 

14. (a) Explain the formation of fringes by transmission gratings.

Consider a transmission grating illuminated normally by monochromatic light.

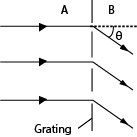
Light is diffracted through the clear space of the grating into a region B where they superpose. Where the resultant path difference of waves through pairs of consecutive slits is an integral multiple of full wavelength; constructive superposition occurs and a bright band is formed.

Where the resultant path difference is an odd multiple of half a wavelength, destructive superposition occurs and a dark band is formed.

(b) Describe how the wavelength of monochromatic light can be measured using a diffraction grating and a spectrometer.

 (c) Explain why an oil layer on the water surface appears colored on a rainy day.

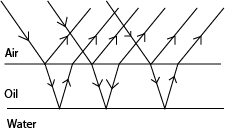
Then white light incident on the oil film, is partly reflected and partly refracted.

Dispersion occurs due to refraction.

The dispersed rays are reflected at oil-water interface.

The light reflected from both surface meet and superpose in air.

Constructive superposition of colored rays give colored fringes. Hence colored oily surface.

(d) Explain

(i) What is meant by plane polarized light

Plane polarized light is one whose electrical vectors varies in only one plane perpendicular to the direction of travel of the wave.

(ii) One application of polarized light.

• Determination of the concentration of sugars
• In sun glasses to reduce glare.

15. (a) What is meant by the following terms as applied to sound?

(i) Resonance

Resonance is said to occur when a system is set to oscillate at own natural frequency as result of impulses received from another system vibrating at the same frequency,

(ii) Fundamental frequency

This is the lowest frequency an instrument can produce.

(b) Describe an experiment to determine end- correction of resonance tube.

(c) A wire of length 50cm, density 8.0gcm^-3 is stretched between two points. If the wire is set to vibrate at fundamental frequency of 15Hz, calculate

(i)The velocity of the wave along the wire

(ii) The tension per unit area of cross section of wire.

(d) Explain using the principle of superposition of wave the formation of

(i)Beats

When two waves of nearly equal frequencies and similar amplitudes are sounded together, they superpose. When they meet in phase constructive interference takes place and loud sound is heard. When they meet completely out of phase, destructive interference takes place and soft sound is heard. A periodic rise and fall in intensity of sound is heard referred to as beats.

(ii) Stationary waves

When two waves of the same frequency, equal wavelength and equal amplitude are propagating in the same medium in opposite direction they meet and superpose; the resultant displacement of particles in medium at any point is the sum of displacements due to each of the waves at that point.

At point where waves meet in phase, the amplitude is maximum. These are antinode. Where the waves meet out of phase a minimum amplitude is formed. The resultant wave is a stationary wave.

16. (a) (i) what is plane polarized light?

Plane polarized light is one in which the variation of electric vector takes place in one plane only.

(ii) Why is it not possible to polarize sound waves?

Sound waves are longitudinal waves whose vibrations are parallel to the direction of propagation of the wave.

(b) (i) Unpolarized light is incident on a sheet of polaroid, A, as shown below

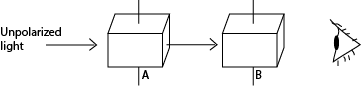
Explain what would be observed if a second polarized sheet B is rotated about an axis perpendicular to the direction of incidence.

Solution

Bright light will be seen or no light will be seen. If the orientation of B is the same as A, bright light is viewed and even when rotated, the bright light remain because the orientation of B does not change its orientation with respect to plane of its polarization

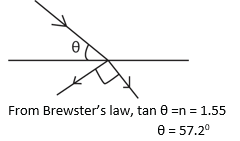
(ii) Sunlight is reflected off a glass window of refractive index 1.55. What should be the elevation of        the sun be if the reflected light is to be completely polarized?

(c) Given the diffraction grating and spectrometer, describe how you would use them to measure the wavelength of light from a given source.

(d) A parallel beam of monochromatic light of wavelength 650nm is directed normally to diffraction grating which has 600 lines per mm. Determine

(i) the number of diffraction image.

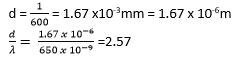
The highest order is 2 and total number of images is 5

(ii) the angle of diffraction of the highest order diffraction image.

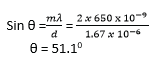
17. (a) (i) Define the terms wave front and a ray in reference to a progressive wave (02mark)

Wave front is a section through an advancing wave along which all particles are in phase 

A ray in the direction of advancing wave.

(ii) Draw a sketch diagram showing reflection of a circular wave by a plane reflector.

 

(b) The figure below shows a wave travelling in positive x- direction away from origin with a velocity of 9ms^-1.

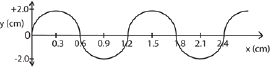
(i) What is the period of the wave? (03marks)

 

 

(c) What is meant by Doppler Effect? (01mark)

This the apparent change in frequency of a wave due to relative motion between the source and observer.

(d) One species of bats locates obstacles by emitting high frequency sound waves and detecting the reflected waves. A bat flying at a steady speed of 5ms^-1 emits sound of frequency 78.0 kHz and is reflected back to it.

(i) Derive the equation for the frequency of the sound waves reaching the bat after reflection (05marks)

(ii) Calculate the frequency of sound received by the bat given the speed of sound in air is 340ms^-1. (02 marks)

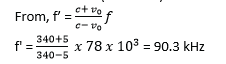
(e) (i) What is meant by intensity of a sound note? (01mark)

This is the rate of flow of energy through an area of 1m² perpendicular to the direction of flow of sound wave.

(ii) Distinguish between loudness and pitch of a sound note. (01mark)

Loudness of sound is dependent on intensity whereas pitch  is dependent frequency, i.e. the higher the frequency the higher the pitch.

18. (a) What is meant by the following terms

(i) Unpolarized light, (01mark)

Unpolarized light is one whose vibrations in electric and magnetic vectors take place in more than one plane.

(ii) Plane polarized light (01mark)

It is the one whose electric vector varies in only one plane.

(b)(i) Describe briefly how plane polarized light is produced by double refraction (03marks)

• 
  • A narrow beam of monochromatic light is made incident on nicol prism and viewed from the opposite side as shown above
  • The angle of incidence is gradually increased or changed and at each angle, the Polaroid is rotated about an axis through its plane.
  • At one point, the emergent light is plane polarized.

(ii) Explain briefly one application of polarized light. (02marks)

• When polarized light is passed through a stressed material, bright and dark fringes are seen on screen which vary according to the concentration of stress. Thus stress analysis.
• In determination of the concentration of sugar in solution, polarized light is passed through the solution and viewed through an analyzer. The analyzer is turned through angle until light is extinguished. This angle is proportional to the concentration

 

 

 

(c) Explain

(i) How two coherent sources are obtained using a biprism (03marks)

• A prism of a very large angle is placed with its refractive edge facing a monochromatic source of light, S.

• Light incident on face L is refracted to appear to come from a point S₁ and that incident on Q appears to come from S₂ due to refraction.
• The two sources are coherent since they originate from the same source S leading to interference where they superpose.

 

(ii) Why interference effects are not observed in thick films with white light (03marks)

But for thick film illuminated normally with white light, each color attains this path difference forming  bright bands. In thick films, these different color bands  overlap  causing uniform illumination and the interference pattern disappear.

(d)  In Young’s double slit experiment, the slits are separated by 0.28mm and the screen is 4m away. The distance between the fourth bright fringe and the central fringe is 1.2cm. Determine the wavelength of light used in the experiment. (04marks)

Solution

(e) Explain the effect of increasing the number of narrow slits in diffraction grating on the intensity of diffraction fringes. (03marks)

Increasing the number of slits, the intensity of the principal maxima increases while that subsidiary maxima decreases. The interference at the principal maxima are always constructive hence intensity increases. Interference at the subsidiary maxima is always destructive, hence the intensity decreases.

19. (a) (i) Define amplitude of a wave. (01mark)

It is the maximum displacement of a particle in a transmitting medium from its equilibrium position

(ii) State two characteristics of a stationary wave (02 mark)

• Amplitude of a wave varies from place to place along the profile.
• Wave energy is not transmitted along the profile.
• At certain points called nodes, the particles are permanently at rest
• Has nodes and antinodes.

(iii) A progressive wave y = asin(ax-kx) is reflected at a barrier to interfere with the incoming wave. Show that the resultant is a stationary wave (04marks)

Incident wave y₁ = asin(ωt – kx)

Reflected wave y₂ = asin(ωt + kx)

Resultant wave = y₁ + y₂

                               = asin(ωt – kx)+ asin(ωt + kx)

= 2asinωtcos kx

= (2acoskx)sinωt

=Asinωt

Where A is the amplitude of a wave

Now A = 2acos kx varies with distance x.

Therefore the wave is a stationary one.

 

(b) (i) What is meant by beats? (02marks)

A beat is the periodic rise and drop in intensity of sound heard when two notes of nearly equal frequency are sounded together.

(ii) Describe how you can determine the frequency of a musical note using beats. (05marks)

(c) Two open pipes of length 92cm and 93cm are found to give beat frequency of 3.0Hz when each is sounding in its fundamental note. If the end errors are 1.5cm and 1.8cm respectively, calculate the

(i) velocity of sound in air (04marks)

(ii) frequency of each note (02mark)

20. (a) (i) Define the term diffraction. (01mark)

Diffraction is the spreading of a wave beyond its geometrical shadow (boundaries) leading to interference.

(ii) What is meant by plane polarized light? (01mark)

Plane polarized light is one in which the variation of its electric vector takes place in one plane only.

(b) (i) Describe how polarized light is produced by double refraction. (05 marks)

• A narrow beam of ordinary light is made incident on nicol prism and viewed through analyzer as shown above
• The angle of incidence is gradually increased or change and at each angle, the polaroid is rotated about an axis through its plane.
• At certain angle of incidence, light is completely extinguished
• At this point, the emergent light is plane polarized.

 

(ii) State two uses of polarized light (02mark)

Used in sunglasses to reduce glare

Used in photo-elasticity to identify regions of stress in a material

Used to determine the concentration of sugars

 

(iii) A parallel beam of unpolarized light incident on transparent medium of refractive index 1.62, is reflected as polarized light. Calculate the angle of incident in air and angle of refraction in the medium (03marks)

 

(c) (i) what is diffraction grating? (01mark)

A diffraction grating is a transparent plate with many small parallel lines drawn on it using a diamond pencil

(ii) Sodium light of wavelength 5.890 x 10^-7m and 5.896 x 10^-7m fall normally on a diffraction grating. If the first order beam, the two sodium lines are separated by 2minutes, find the spacing of grating (03marks)

(d) State three differences between the spectra produced by a prism and that by diffraction grating. (03marks).

 

Prism	Diffraction grating
– Produce single spectrum at a time	– Many spectra at a time
–   Shorter wavelengths are deviated most	– Longer wavelength are deviated most.
–    Produce less pure spectrum	–          Produce more pure spectrum

If the air is restricted in volume, ρ is constant so that P T. from the above expression, speed of sound in air increases with increase with temperature.

(c)(i) A train moving with uniform velocity, v₁ sounds a horn as it passes a stationary observer. Derive expression for the apparent frequency of sound detected by the observer. (03marks)

(d) Describe a simple experiment to show interference of longitudinal waves. (04marks)

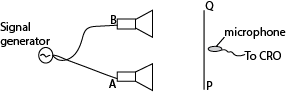
Two loud speaker A and B were connected to a signal generator. A microphone connected to the y-plates of s C.R.O with the time base switched off.

As the microphone is moved along the line PQ which is parallel to the line joining the loud speaker, the vertical trace on the screen of CRO is seen to increase to maximum and reduce to minimum at equal distances. The alternative maximum and minimum intensity are interference pattern.

Or

Tube A is fixed while B is free to move. A note is sounded at S and detected at E. Tube B s then pulled out slowly. It is noted that the sound detected E increases to a maximum and reduces to minimum intensity at equal intervals of the length of the tube. The alternate maximum and minimum intensity formed are interference pattern.

 

22. (a) State three differences between mechanical and electromagnetic waves (03marks)

 

Mechanical wave	Electromagnetic waves
–  Need material medium for propagation –  Propagate at relatively low speed –   Have longer wavelength –   Are due to vibration or oscillation of particles in transmitting medium.	–     Can propagate in vacuum –     Propagate as very high speed –     Shorter wavelength –    Are due to vibration in electric and magnetic field

 

(b)(i) State the principal of superposition of waves (01mark)

The resultant displacement at any point is the sum of displacements due to the separate waves.

(ii) Explain how interference pattern is formed (03marks)

When two waves from coherent source sources cross they superpose. Where the path difference is odd multiple of a half a wavelength, cancellation occurs resulting in minimum intensity.

Where the path difference is equal to integral multiple of a full wavelength, reinforcement occurs resulting in maximum intensity. This leads to formation of alternate permanent regions of maximum and minimum intensity called interference.

(c)

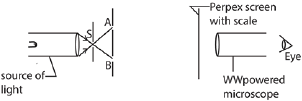
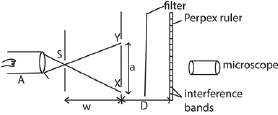
A source of light, a single slit, S, and a double slit (A and B) are arranged as shown in figure 2.

(i) Describe what is observed on the screen through the microscope when white source of light is used. (02marks)

Sets of numerous colored fringes are seen on screen. The central fringe is white with colored fringes on either side. For each set, blue fringe is nearest to the central one while red is farthest.

(ii) How would you use the set up above to compare the wavelength of red and blue light? (04marks)

A source of white light is used. A red filter is placed in front of the slits. The number of bright fringes in fixed length on the screen is counted and fringe separation, y_r is determined. The red filter is replaced by a blue filter and experiment repeated and fringe separation y_b is determined. It is found y_r> y_b

Since fringe separation y is proportional to wave length, from y =

It implies that the wavelength of red light is greater than wavelength of blue light.

(iii) Explain what is observed when slit, S, is gradually widened. (03marks)

Fringes gradually disappear. A large slit S is equivalent to many narrow slits that the fringes overlap to form uniform illumination.

(d)  A diffraction grating of 500 lines per mm is illuminated normally by light of wavelength 5.26 x 10^-7m. Find the total number of images seen. (04marks)

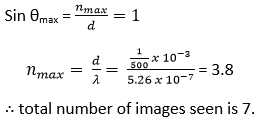
23. (a) (i) What is a wave? (01mark)

A wave is a periodic disturbance that travels with a definite velocity through the medium.

(ii) Explain why an open tube is preferable as a musical instrument. (03marks)

A closed pipe gives only odd numbered harmonics while an open pipe produced both odd and even numbered harmonics. Hence open pipe gives many different overtones and thus better quality musical notes than closed pipes.

(b)(i) State two factors that affect the speed of sound in air (01mark)

• Temperature of the air
• Humidity of the air
• Wind velocity

(ii) Explain the term reverberation. (02mark)

Reverberation occurs when sound produced at one instant by a source is closely followed by its echo. This is due to the reflecting surface being too close to the source. Therefore the observer fails to distinguish between the original sound and its echo; the observer gets the impression that the original sound is prolonged.

 

(iii) What is the implication of reverberation in a hall? (02marks)

The soft clothing of the audience absorb the sound instead of reflecting it. Consequently, the music and speed appear to be weaker.

Reverberation of a small degree is necessary because it enhance audibility. However, if it is too long, it makes music and speech sound confused and indistinct.

(c) Describe an experiment to determine the velocity of sound in air using resonance method. (06mark)

(d) (i) What is harmonic in sound? (01mark)

Harmonic is one of the frequencies that cam be produced by a given instrument (both stringed and piped)

 

(ii) A string of length 0.5m and mass 50g is stretched between two fixed points. If the tension in              the string is 100N, find the frequency of second harmonic. (04marks)

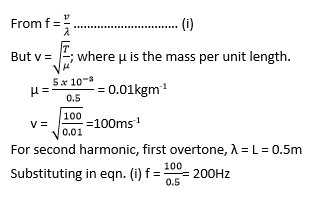
24. (a) Distinguish between constructive and destructive interference. (03marks)

Constructive interference occurs when two waves superpose in phase whereas destructive interference occurs when two waves are superposed out of phase.

(b) (i) Explain how interference fringes formed in air-wedged film between to glass slides when monochromatic light is used. (06marks)

Solution

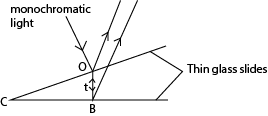
Light from monochromatic source incident on the wedge at O  is partially reflected upwards from the bottom of the top glass slide and partially transmitted through the air film at O to point B where it is reflected upwards.

The two wave trains are coherent and when they superpose above the upper side, they produce interference pattern in form of alternative dark and bright fringes parallel to the line of intersection of the slide.

The path difference is 2t where t is the small thickness of the film at O.

At C where the path different is apparently zero, a bright fringe is expected, however, a dark fringe is observed. This is due to phase change of π radians (equivalent to path difference of (λ/2) that occurs when a wave is reflected at a denser medium.

(ii) Describe the appearance of fringes when white light is used. (02marks)

Colored fringes are observed.

 

(c) Two glass slides in contact at one end are separated by a sheet of paper 15cm from the line of contact to form an air-wedge. When the air wedge is illuminated normally by light of wavelength 6.0 x 10^-7m, interference fringes of separation 1.8mm are found in reflection. Find the thickness of the paper. (04marks).

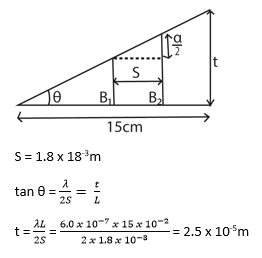
(d) (i) Describe, with aid of a labelled diagram, one method of producing plane polarized light. (04marks)

Solution

• A narrow beam of ordinary light is made incident on nicol prism and viewed through analyzer as shown above
• The angle of incidence is gradually increased or change and at each angle, the polaroid is rotated about an axis through its plane.
• At certain angle of incidence, light is completely extinguished
• At this point, the emergent light is plane polarized.

 

Or

• A narrow beam of unpolarized light is made incident on transparent medium.
• The reflected light is observed through a Polaroid.
• The angle of incidence is varied
• At each angle of incidence, the polaroid is rotated about the axis along the light incident on it
• At one angle of incidence, the light gets cut off the observer as the Polaroid is rotated.
• The reflected ray is now completely polarized.

(ii) State two uses of polarized light. (01mark)

• Determination of sugar concentration
• Stress analysis in some materials like glass

 

25. (a) State three differences between sound and light waves. (03marks)

Mechanical wave	Electromagnetic waves
–  Need material medium for propagation –  Propagate at relatively low speed –   Have longer wavelength –   Are due to vibration or oscillation of         particles in transmitting medium.	–    Can propagate in vacuum –     Propagate as very high speed –     Shorter wavelength –    Are due to vibration in electric and           magnetic field

 

(b) Distinguish between free and damped oscillation? (02marks)

Free oscillation	Damped oscillation
–          Amplitude is constant –          Wave energy is constant	–          Amplitude decreases with time –          Wave energy decreases with time

 

(c) (i) What is meant by resonance? (02marks)

Resonance occurs when the forcing frequency in an oscillation is equal to natural frequency of the oscillating (vibrating) body. The body then oscillates (vibrates) with maximum or constant amplitude.

(ii) Describe with aid of diagram, an experiment to investigate the variation of frequency of stretched string with length. (06marks)

(d) (i) Calculate the frequency of beats heard by stationary observer when a source of sound of frequency 80Hz is receding with a speed of 5.0ms^-1 towards a vertical wall. (speed of sound in air = 340ms^-1) (05marks)

Solution

 

(ii) State two uses of beats. (02marks)

• Tuning musical instrument to a desired note
• Determining the unknown frequency of a note

 

26. (a) State Huygens’ Principle. (01 mark)

Every point on a wave front may be regarded as a source of secondary spherical wavelets which spread out with the wave velocity. The new wave front is the new envelope of these secondary wavelets.

 

(b) Monochromatic light propagating in air is incident obliquely onto a plane boundary with a dielectric of refractive index, n.

(i) Use Huygens’ principle to show that speed, V, of light in the dielectric is given by

V = (c/n)  where c is the speed of light. (06marks)

 

Consider a beam of parallel rays between LO and MD incident on the plane surface of the medium from air in the direction shown, and suppose that a plane wave front has reached the position OA at a certain instant,

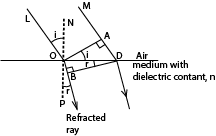
In figure above, each point between O, D becomes a new centre of disturbance as the wave front advances to the surface of the medium, and the wave front changes in direction when the disturbance enters the medium.

Suppose that t is the time taken by the light to travel from A to D. The disturbance from O travels a distance OB, or Vt, in the medium in a time t, where v is the velocity of light in medium.

At the end of the time t, the wave fronts in the medium from the other secondary centres between O, D reach the surfaces of spheres to each of which DB· is a tangent.

Thus DB is the new wave front in the water, and the ray OB which is normal to the wave front is consequently the refracted ray.

Since c is the velocity of light in air, AD = ct.

Now

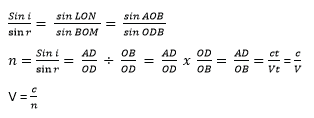
(ii) If the wavelength of the light is 600nm in air, what will it be in a dielectric of refractive index 1.50? (04marks)

 

 

 

(c) (i) What is meant by interference of waves? (01mark)

It is superposition of waves resulting into alternate regions of maximum and minimum intensity.

(ii) State the conditions necessary for interference fringes to be observed, (02mark)

• The wave trains must have nearly the same or equal amplitudes.
• There must be a constant phase relationship between the two wave trains.
• The source must be very close to each other.
• The screen must be very as far as possible from the sources.

(iii) Explain the term path difference with reference to interference of two wave-motions.

Path difference in difference in distances travelled by two wave trains from secondary sources to the point of overlap. Where they meet, the two waves superpose leading to reinforcement or cancellation. Where the path difference is an integral multiple of a wavelength, constructive interference takes place. Where the path difference is an odd multiple of half a wave length, destructive interference takes place.

 

(d) Two glass slide in contact at one end are separated by a wire of diameter 0.04mm at the other end to form a wedge. Fringes are observed when light of wavelength 5.0 x 10^-7m is incident normal to the slides. Find the number of fringes which can be observed. (03marks)

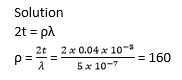
27. (a) (i) What is meant by amplitude and wavelength as applied to wave? (02marks)

Amplitude is the maximum displacement of a particle in the transmitting medium from the mean position.

Wavelength is the distance between two successive crests or troughs in a wave or the distance between two adjacent particles in the medium which are n phase for a progressive wave.

 

(ii) State differences between progressive and stationary waves

(b) The displacement, y, of a wave travelling in the x- direction is given at time, t, by

(c) (i) What is meant by the terms overtones and beats? (03marks)

Overtones are notes of higher frequency than the fundamental frequency for a particular instruments.

Beats are periodic rise and fall in intensity of sound heard when two notes of nearly equal frequencies and similar amplitudes are sounded together.

(ii) State two uses of beats (02marks)

• Measurements of frequency of a note
• Tuning a musical instrument to a given note

(iii) A tube 1m long closed at one end has its lowest resonance frequency at 86.2Hz. With a tube of identical dimensions but open at both ends, the first resonance occurs at 171Hz.

Calculate the speed of sound and the end correction. (06marks)

28. (a)(i) State the superposition principle as applied to wave motion. (01mark)

When two waves meet in a medium, the resultant displacement is the sum of the displacements due to the individual waves.

(ii) What is meant by optical path? (01mark)

Optical path is the length in a medium that maintains the same number of waves as a given length in a vacuum.

Or

It is the product of the refractive index of a medium and the geometrical path length in air or vacuum.

(b) (i) state the conditions which must be satisfied in order to observe an interference pattern due to two waves (02marks)

• For observable interference pattern, the source should have equal amplitude and constant phase relationship.
• The colors/source should be close to one another.

(ii) Explain why an oil film on a water surface appears to be colored. (04marks)

Colors on an oil film on water are due to interference of light.

When light from the sky reaches the oil film, it is partly reflected and partly refracted.

The refracted light is dispersed into colors is totally internally reflected on oil-water boundary.

The two reflected waves superpose when they meet.

The colors for the waves that meet in phase are seen while those that meet out of phase are missing.

 

(c)

The figure above shows an experiment set up to demonstrate Young’s interference fringes. Explain what is observed when the

(i) Slit X is covered. (02marks)

Interference patterns disappear and uniform illumination is observed on the screen. Since there is only one source, interference does not take place.

(ii) Slit S is widened (02marks)

The bands gradually disappear. This is equivalent to many slits causing overlap and hence uniform illumination.

(iii) Separation, a, of the slits X and Y is reduced keeping w fixed. (02marks)

The separation of fringes increase

(iv) Distance, w, is reduced. (02 marks)

The separation of the fringes remain the same but the fringes become brighter. The smaller the distance the greater the intensity of light.

(c) Monochromatic light of wavelength 600mm is incident normally on a plane diffraction grating which has 500 lines per mm. calculate the

(i) Number of maxima observed

(ii) Angular position of the first diffraction maximum (02marks)

For additional questions in waves A-level and answers download PDF below

Waves (A-level questions and answers

Thank you so much

Please share with a friend

Compiled by Dr. Bbosa Science