Optical instruments

Optical instruments

An optical instrument is a device that aids vision working on the principles of reflection and refraction of light.

Examples are the human eye, microscope, telescope, projector and lens cameras.

Terminology in optical instrument

1. Near point.

This is the nearest position that can be focused distinctly by the unaided eye. The distance from the eye to the near point is known as the least distance of distinct vision (LDDV), and it is usually denoted by D.

 

The least distance of distinct vision of a normal eye is 25cm.

2. The far point

This is the furthest point which can be seen distinctly by the unaided eye. For normal eye, the farthest distance is infinity.

3. Accommodation

This is the ability of the eye to clearly see near and far object.

4. Visual angle (α)

This is the angle subtended by the object at the unaided eye.

5. Angular magnification (magnifying power, M)

This is the ratio of the angle subtended at the eye by the image when using an instrument to the angle subtended at unaided eye by the object.

Note that the height of the image formed by the eye on the retina is proportional to the angle subtended at the eye by the object. (I.e. the greater the visual angle, the greater is the apparent size of the object).

 

The mammalian eye

This an organ that helps us to see

Parts of the eye

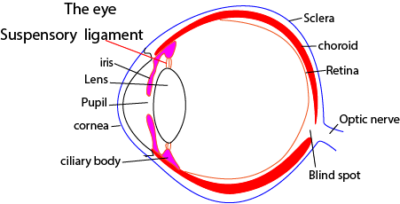
The iris controls the size of the pupil

The pupil allows in light into the eye

Ciliary body regulate the size of the lens

Cornea and lens refract light to the retina where the image forms

Sclera protects and maintains the shape of the shape of the eye.

Accommodation

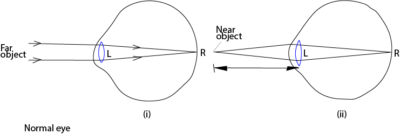
This is the ability of the eye focus objects at different distances on the retina

To view near object the ciliary muscle contract creating less tension on suspensory ligaments the lens fattens and its refractive power increases.

To view distant objects the ciliary relax creating a tension in the suspensory ligament, the lens this reducing its refractive power.

Eye defects

• Short sight/myopia

A person with short sightedness does not see distant objects clearly because rays of light from a distant object are focus in front of the retina

 

Short sightedness is caused by too long eye-ball or to strong lens

 

Short sightedness is corrected using concave lens

• Long sight/hyper myopia

A person with long sightedness does not see near objects clearly because rays of light from a near object are focus behind of the retina

 

Long sightedness is caused by too short eye-ball or to weak lens

Long sightedness is corrected using convex lens

 

2. Microscope

These are used to magnify near objects when in normal use. The image formed by microscope is at the least distance of distinct vision from the eye.

Types of microscope

• Simple microscopes (magnifying glasses)
• Compound microscope

 

 

Simple microscope

A magnifying glass consists of a converging lens which forms a virtual, upright image of the object placed inside its focal point.

• Final image in normal adjustment

Consider an object subtending an angle α at the eye when the object is at the near point.

tanα ≈ α for small angle in radians

α =  …………………………………. (i)

 

If a convex lens is used to view the object

For small angle tanα’ ≈ α’ for small angle in radians

α’ =  …………………………………. (ii)

Linear magnification from (i) and (ii), m =  =  =

 

From  and multiplying through by v

 

Since, v = D

or m =  -1 where f is the focal length

 

• Final image at infinity

For the image to be formed at infinity, the object must be at, f.

Using unaided eye

 

tanα ≈ α for small angle in radians

α =  …………………………………. (i)

 

For small angle tanα’ ≈ α’ for small angle in radians

α’ =  …………………………………. (ii)

Linear magnification from (i) and (ii), m =  =  =

 

From  and multiplying through by v

 

Since, v = f

or m =  -1 where f is the focal length

It should be noted that higher magnification is obtained from a lens of short focal length.

Compound microscopes

• These utilize two converging lenses of short focal lengths are used.
• The lens closer to the object is called objective lens while that through which the final image is viewed is called the eyepiece.
• When the microscope is used, the object O is placed at a distance slightly bigger than the principal focus, F, of objective lens, to form an inverted image I₁.
• I₁ the a real object of the eye piece forms between its principal focus F₀ and its optical center so that a large virtual image I₂ is viewed by the eyes at least distance D.

 

 

For an aided eye (using the instrument)

For small angle, tanα’ ≈ α’ for small angle in radians

α’ =  ………………………………………… (i)

For unaided eye

α =  ………………………………………….. (ii)

Magnifying power, m =  =  =

Or

Linear magnification can also be expressed as, m =  = m_e x m_o.

Where, m_e and m_o are linear magnifications of the eye piece and objective lenses respectively.

m_o  =  – 1 and me =  – 1

 

m = (  – 1)(  – 1)

 

Examples 1

The objective of a compound microscope has a focal length of 2cm while the eye piece has a focal length of 5cm. an object is paced a distance of 2.5cm in front of objective lens. The distance of the eye piece from the objective is adjusted so that the final image is 25cm in front of the eyepiece. Find the separation of the lenses.

Solution

u₀ =2.5cm, f₀ = 2.0cm, v₀?,

v_e = D = -25cm, u₂ ?

For objective lens

 

=> v₀ = 10cm

 

 

=> u_e = -4.2cm

= 10 – ^–4.2 = 14.2cm

 

Example 2

The objective and eye piece of a compound microscope have focal lengths of 1cm and 5cm respectively. the distance of lens separation is 25 cm. The object is placed1.05cm from the objective lens.

 

• Find the position, and nature of the final image
• Calculate the magnification

Solution

In objective lens

 

; v_o = 21

= 4cm, f_e = 5

; v_e = -20cm

 

Nature of image, virtual, magnified and inverted.

 

(b) Magnifying power, m = (  – 1)(  – 1) =80

Microscope with image at infinity

To form the final image at infinity, the image of the objective lens must be formed at the principal focus. F_e, of eyepiece

 

 

 

For unaided eye (using the instrument)

For small angle, tanα’ ≈ α’ for small angle in radians

α =  ………………………………………… (i)

For aided eye

α’ =  ………………………………………….. (ii)

Magnifying power, m =  =  =

But  is the linear magnification of objective lens

m_o =  =

m = (  – 1)

NB: D is always 25cm for this case

Example 3

An object is placed at a distance of 5cm from the objective of focal length 4cm. if the eye piece has focal length of 15cm and final image is formed at infinity, calculate

• Separation of the lens
• Final magnification of the microscope.

 

Solution

For objective lens, u =5cm, f₀ = 4cm, v =?

From

, vo = 20cm

Separation of lenses = v_o + f_e = 20 + 15 = 35cm.

(ii) D is always 25cm

Magnification, m = (  – 1)   = (  =6.7

Telescopes

These are instruments focus the light and make distant objects appear brighter, clearer and magnified

They are mainly divided into two

• Refractive telescope
• Reflective telescope

Refractive telescope

These are telescopes which use lenses and they include

• Astronomical telescope
• Terrestrial telescope
• Galilean telescope

Astronomical telescope

This consists of two convex lenses: the objective of a long focal length and eye-piece of short focal length. The objective forms a real diminished image of a distant object at its focal length.

• Astronomical telescope in normal adjustment (image at infinity)

Note,

• when an astronomical telescope is in normal adjustment, the final image is formed at infinity
• the focal length of the objective is bigger than that of eye piece
• the principal focuses of the two lenses coincide

To obtain the magnification, m, we assume the eye is very close to the eye piece.

For an aided eye (using the instrument)

For small angle, tanα’ ≈ α’ for small angle in radians

α’ =  ………………………………………… (i)

Where, h, is the height of image I, f_e is the focal length of eye piece

For unaided eye

α =  ………………………………………….. (ii)

f₀ is the focal length of objective lens

Combining equations (i) and (ii)

Magnifying power, m =  =  =

Thus the angular magnification is the ratio of the focal length of objective lens to the focal length of the eyepiece.

Note that, bigger magnification power is obtained when the focal length of objective lens is very big compared to the focal length of eye piece.

Secondly the separation of the lenses is the sum of the focal length of the objective and eye-piece lenses, (f₀ + f_e).

 

 

• Astronomical telescope with final image at near point

When the image of a telescope forms an image at near point, the telescope is not in normal adjustment, the eye is accommodated, although the image is still clearly seen. The diagram below illustrates the formation of the final image.

 

 

 

 

 

 

 

 

The objective lens forms a real image of the distant object at its the principal focus F₀, and the

eye piece is moved so that the image is nearer to it than its focus, F_e, thus acting as a magnifying glass. The separation of the lens is (f_o + u).

To obtain the magnification, m, we assume the eye is very close to the eye piece.

For an aided eye (using the instrument)

For small angle, tanα’ ≈ α’ for small angle in radians

α’ =  ………………………………………… (i)

Where, h, is the height of image I, f_e is the focal length of eye piece

For unaided eye

α =  ………………………………………….. (ii)

f₀ is the focal length of objective lens

combining equations (i) and (ii)

Magnifying power, m =  =  =

Since  is  linear magnification

 = m_e =

 =

Note that the magnifying power of astronomical telescope in normal use is greater than when in its normal adjustment.

Eye ring

The eye-ring the best position for the eye, is the circular image of the objective in the eye-piece.

The figure below illustrates three rays from a point on the distant object which pass through the objective, forming an image at Y.

The position of the eye ring, M, can be calculated as follow position as

C₁C₂ = f₁ +f₂

The focal length of eye piece, L₂ = f₂

From

The distance C₂M or v is given by

 

 

=

=

=

But angular magnification of telescope in normal adjustment =

Thus anglar magnification, M =

Advantages of refractive astronomical telescope

• It is less bulky since it is more compact
• Refractor telescopes are rugged. After the initial alignment, their optical system is more resistant to misalignment than the reflector telescopes.
• The glass surface inside the tube is sealed from the atmosphere so it rarely needs cleaning.
• Since the tube is closed off from the outside, air currents and effects due to changing temperatures are eliminated. This means that the images are steadier and sharper than those from a reflector telescope of the same size.

Disadvantages of astronomical telescope to view objects on earth

• form inverted images

Example 4

An astronomical telescope consists of two thin converging lenses of focal length 100m and 10cm respectively. if the final image of a distant object is virtual and is 20cm from eyepiece. Find the separation of the lenses.

Solution

f₀ = 100cm, f_e=cm, v = -20cm

From,

, u = 7cm

Separation = f_e + u = 100 + 7 = 107cm

Example 5

• What is the meant by the following as applied to a telescope
• Magnifying power

The ratio between the dimensions of the image and the object.

• Eye ring

This is the ideal position of the eye for observing the image through the telescope.

• (i) Draw a ray diagram to show how the formation of the final image by an astronomical telescope in normal use.

(ii) With the aid of the diagram in (b)(i), derive an expression for the magnifying power of astronomical telescope in normal use.

(iii) Give the disadvantage of the telescope in (b)(i) when used to view distant object on earth. Describe how the telescope can be modified to overcome this disadvantage.

 

Disadvantage: it forms inverted image. This can be overcome by using an erect lens or converting it into a terrestrial telescope.

 

 

• Find the separation of eyepiece and objective of astronomical of magnifying power 20 and in normal adjustment if its eyepiece has a focal length of 5cm

Solution

Separation = f₀ + fe

m = 20, f_e = 5cm

but m =

20 =

f_o  =100cm

 

Separation = 100 +5 = 105cm

 

Terrestrial telescope

This uses one more convex lens than astronomical refracting telescope. It has an erecting lens of focal length, f, placed between the objective and the eyepiece lens.

The erecting lens is positioned such that image, I, of the object is formed at 2f.

The terrestrial telescope is longer than the astronomical telescope by 4f; where f is the focal length of the erecting lens.

Angular magnification, m =

It is used to view different objects on the earth surface

The objective lens forms a real image I1

Action

• The objective lens forms a real image I₁ of the distant object at its principal focus, F_o. which is at 2f of erecting lens L
• The erecting lens L form an erect image I at its 2f and at principal focus, Fe, of the eye piece. The images I₁ and I have equal sizes
• The eye piece forms a virtual image at infinity.
• The angular magnification of terrestrial and astronomical telescopes is the same in the same adjustments.

Advantages of a terrestrial telescope

It forms upright images

Disadvantages of a terrestrial telescope

• Long and bulky
• Extra diffraction occurs in erecting lens reducing the clarity of the final image

Galilean telescope

It consists of two lenses, the objective which is converging lens of long focal length and the eye piece which is a diverging lens of short focal length. It is intended to produce an erect final image. Always the final image produced by the objective beyond the eyepiece.

The separation of the lenses is equal to the difference in the magnitude of focal lengths, i.e. (f₀ – f_e).

• Galilean telescope in normal adjustment (final image is at infinity)

 

Light from distant object incident on objective lens is refracted to form real inverted image at its principal focus f₀. In normal adjustment, the principal focuses of both lenses coincide. The eye piece therefore forms the final image of the object at infinity.

To obtain the magnification, m, we assume the eye is very close to the eye piece.

m =

For small angles, α and α’ are measured in radians

α ≈ tan α =  and α’ ≈ tan α =

Substituting for α and α’

Magnifying power, m =  =  =

 

• Galilean telescope not in normal adjustment (final image at near point)

 

To obtain the magnification, m, we assume the eye is very close to the eye piece.

For an aided eye (using the instrument)

For small angle, tanα’ ≈ α’ for small angle in radians

α’ =  ………………………………………… (i)

Where, h, is the height of image I, f_e is the focal length of eye piece

For unaided eye

α =  ………………………………………….. (ii)

f₀ is the focal length of objective lens

 

Combining equations (i) and (ii)

Magnifying power, m =  =  =

Since  is linear magnification

 = m_e =

 =

Advantages of a Galilean telescope

It forms a final erect image.

It is shorter than the terrestrial and astronomical telescopes because the separation of lenses is

f₀-f_e.

 

Disadvantages of a Galilean telescope

It has a small field of view.

It has a virtual eye ring not accessible to the observer.

 

Example 6

A convex lens of focal length 60m is arranged coaxially with a diverging lens of focal length 5cm to view a distant star. Calculate the magnifying power if the image of the star is formed at a distance of 25cm in front of the eyepiece.

Solution

f₀ = 60cm, f_e = -5cm, D -25cm

From, m =  =  = 9.6

Eye ring of a telescope [Exit pupil]

This is the best position where the eye can be placed so as to receive all rays that are refracted by the objective lens

Reflecting telescope

In these telescopes, a concave mirror of long focal length is used instead of convex lenses as objectives.

They include

Advantages of reflecting telescopes

(i) There is no chromatic aberration since no refraction occurs at the objective

(ii) There is no spherical aberration since a paraboloidal mirror is used.

(iii) It is cheaper to construct since only one surface requires grinding.

(iv)When curved mirrors of large diameter are used, a greater resolving power is obtained.

 

The prism binoculars

It consists of a pair of a refracting astronomical telescope with two reflecting prisms between each objective and eye piece

Action

• The objective lens forms a real inverted of a distant object at its principal focus in from of prism A
• By total internal reflection, the prism A causes a lateral inversion direction through 180⁰.
• The right angled prism B, then inverts the image such that an erect image is formed in front of the eye piece.
• The eye piece then forms a virtual magnified image of the object.

The lens camera

It consists of a lens system, a focusing device, an exposure system and light sensitive film.

Lens camera

• The lens focused light from the object on to film
• The diaphragm controls the amount of light reaching the film
• The shutter controls the exposure time of light reaching the film
• Film contain photosensitive chemicals and is where the image is stored.

 

 

Projection lantern

A projector is an instrument used to produce a large image of a small object.

• The slide, or film, is placed behind the projectorlens outside its focal length and is illuminated buy a small but powerful source of light from concave reflector through condensing lenses.
• A magnified, real and inverted image on the screen

 

 

 

Area magnification = (Area of the image )/(area of the object)

Elimination of chromatic aberration in images formed by magnifying lens

In the lens the light rays from the object are dispersed to form colored images I_b and I_r due the different refractive indices; but when the eye is placed close to the lens, the mages subtend the same angle to the eye. The images overlap reducing chromatic aberration