Geometrical optic (light) revision questions and answers

Geometrical optic revision questions and answers

1. (a) (i) derive the relationship, for convex lens, where u is the object distance, v is the image distance and f is the focal length of the lens. (04marks)

 

Solution

Consider in each case a ray incident on the same lens at a small height h above the principal axis as shown:

 

(ii) State three possible reasons under which an image of real object may not be formed by a convex lens on the screen.  (03marks)

• When the object is placed between the lens and optical center.
• When the distance between the object and the screen is four times the focal length of the lens
• When the object is at the principal focus
• When the screen is between the lens and its principal focus.

(b) Describe an experiment to determine the focal length of a convex lens fixed inside a short cylindrical tube (05marks)

(c) A convex lens of focal length 10cm is arranged coaxially with a concave lens of focal length 18cm. the lens system is used to focus an object placed 24cm from the convex lens on the side remote from the concave lens. the final image is formed on a screen placed 18.6cm from the concave lens. Calculate the;

(i) separation between the lenses. (05marks)

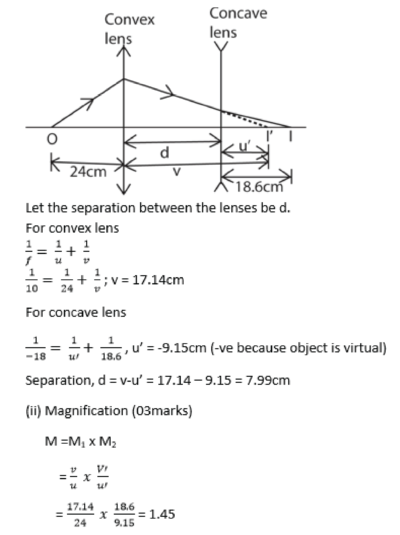
2. (a) (i) State the laws of refraction of light. (02marks)

• The incident ray, refracted ray and the normal at the point of incidence all lie in the same plane
• The ratio of the angle of incidence to the sine of angle of refraction is constant for a given pair of media.

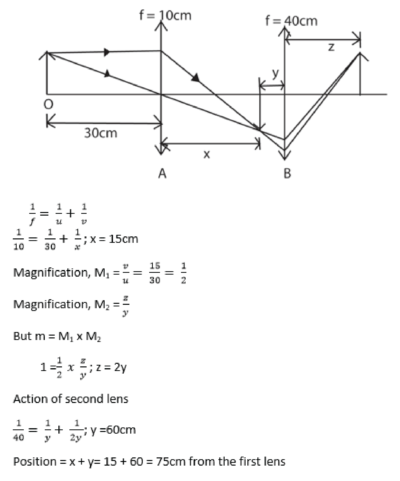
(ii) A small object is placed at a distance of 30.0cm from a converging lens of focal length 10.0cm. Calculate the distance from the first lens where a second converging lens of focal length 40.0cm must be placed in order to produce an erect image of the same size as the object. (05marks)

(b) (i) Draw a diagram to show the formation of an image by a compound microscope in normal adjustment and use it to derive an expression for the magnifying power. (06marks)

 

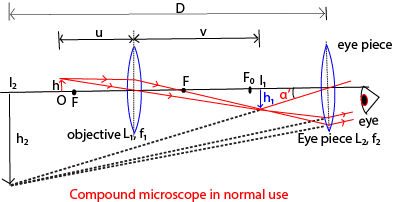
(ii) A microscope has an objective of focal length 10.0cm and eye piece of focal length 20.0cm. If the distance between the objective and eye piece is 20 cm, calculate the magnifying power of the microscope. (03marks)

 

(c) What is meant by the following:

(i) total internal reflection (01mark)

Total internal reflection is the bouncing back of all incident light into the more dense medium when the angle of incidence exceeds the critical angle for a ray originally travelling from the more dense to a lens dense medium.

(ii) critical angle (01mark)

Critical angle is the angle of incidence in more optically denser medium for which the angle of refraction in the less dense medium is 90⁰.

(d) Briefly explain why an observer sees a spectrum of colors through rain drops when it is raining on a sunny day. (02marks)

White light from the sun undergoes dispersion as it enter into the rain drops of water in the sky. Total internal reflection takes place at the opposite side of the rain drop and different colors emerge from the raindrop after refraction. Hence the observer sees a spectrum of colors through raindrops when it is raining on sunny day.

 

 

3. (a) Define the following as applied to telescope

• Eye-ring (01marks)

Eye-ring of a telescope is the image of objective formed by the eyepiece.

• Magnifying power(01mark)

Magnifying power is the ratio of the angle subtended by the final image at the eye when using the telescope to the angle subtended by the object at unaided eye.

(b) What is the significance of the eye-ring of an astronomical telescope? (01mark)

It is the best position for best view in  a telescope

(c) State two advantages of a reflecting telescope over a refracting telescope (02marks)

• there is no chromatic aberration
• there is no spherical aberration
• it is relatively cheap since only one face of the objective needs grinding
• Has high resolving power since the objective may have a high field of view.
• The image is brighter.

 

(d) The figure below shows an optical system consisting of two thin converging lenses arranged coaxially. Lens A has a focal length of 40mm and lens B has a focal length of 375mm. an object O of height 5mm is placed 50mm from A. I₁ is the real image of O and I₂ is the virtual image of I₁ in B and is 250mm from B.

(i) Determine the value of distance, Y of image I₁ from lens A (02marks)

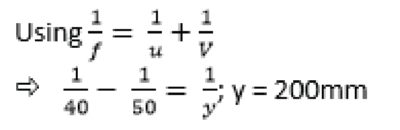
(ii) Calculate the distance, x, between the images I₁ and I₂. (02marks)

(iii) Find the linear magnification produced by the lens system. (02marks)

(e) Name one defect of the image formed by a lens and explain how the defect is minimized in practice. (03marks)

(i) Chromatic aberration can be minimized by placing the eye very close to the lens. This insures that images due to different colors subtend the same angle at the eye. Or by use of chromatic doublet in which the dispersion produced by one lens is reversed by another.

Or

(ii) Spherical aberration can be minimized by using a stopper which allows only central rays to form a sharp image. Or by grinding the lens to suitable shape. Or using a narrow aperture

(f) Explain the following

(i) total internal reflection (03marks)

If a ray of light is incident from a denser to less dense medium, there is partial reflection and partial refraction. When the angle of incidence exceeds the critical angle, all the incident light is reflected back into the denser medium. This is called total internal reflection.

(ii) Formation of mirages (03marks)

On a hot day, layer on air near the ground are hotter and lens dense than layers above. This leads to total internal reflection of rays of light from the sky. And mirage is the image of the sky to the eye by total internal reflection

4. (a) State the laws of refraction of light. (02marks)

• The incident ray, the refracted ray and the normal at the point of incidence all lie in the same plane
• The ratio of the sine of angle incidence to the angle of refraction is constant for a given pair of the media.

(b) Derive an expression for refractive index of a prism in terms of the refractive angle A and the angle of minimum deviation D. (05marks)

(c) A ray of light is refracted through a prism in a plane perpendicular to its edge. The angle of incidence is 30⁰ and the refractive index of the prism is 1.50. Calculate the angle of the prism such that the ray does not emerge when it strikes the second face. (05marks)

 

(d) (i) Describe with the aid of a labelled diagram, the structure and operation of projection lantern. (04marks)

Projection lantern

A projector is an instrument used to produce a large image of a small object.

• The slide, or film, is placed behind the projector lens outside its focal length and is illuminated buy a small but powerful source of light from concave reflector through condensing lenses.
• A magnified, real and inverted image on the screen

(ii) A projector produces an image of area 2m² on a screen placed 5m from the projection lens. If the area of the object slide is 8cm², calculate the focal length of the projection lens. (02marks)

5. (a) (i) State two differences between real and virtual images (02marks)

A real image is formed by actual intersection of rays and can be formed on a screen while virtual image is formed by apparent intersection of rays and cannot be formed on a screen.

(ii) Explain with the aid of a diagram how thick plane mirror forms multiple images (04marks)

Formation of multiple images in thick plane mirror

Multiple images are formed due to partial reflection and refraction at the non-silvered surface of the mirror.

• İmage I₁ is formed by reflection on the glass surface P
• The image I₂ (the brightest is formed by reflection of the most light on the silvered surface Q
• Others by partial refraction

(b) A convex mirror forms a real image which is three times the linear size of the object. When the object is displaced though a distance y, the real mage formed is four times the linear size of he object. If the distance between the two image positions is 20 cm, find the

(i) focal length of the mirror (03marks)

 

(d) Explain how mirage is formed. (03marks)

On a hot day, layer on air near the ground are hotter and lens dense than layers above. This leads to total internal reflection of rays of light from the sky. And mirage is the image of the sky to the eye by total internal reflection

 

7. (a) Define the following as applied to a converging lens;

(i) Principal focus (01mark)

The principal focus of converging lens is the point on the principal axis to which paraxial rays converge after refraction from the lens.

(ii) center of curvature (01mark)

The center of curvature of lens is the center of the sphere of which one of the spherical surfaces of the lens form part.

(b) Find the power of a lens of focal length 15 cm (02marks)

(c) Derive an expression for focal length of a lens in terms of the radii of curvature of its surfaces and refractive index (05marks)

 

Consider  a ray parallel to the principal axis, incident at height, h.

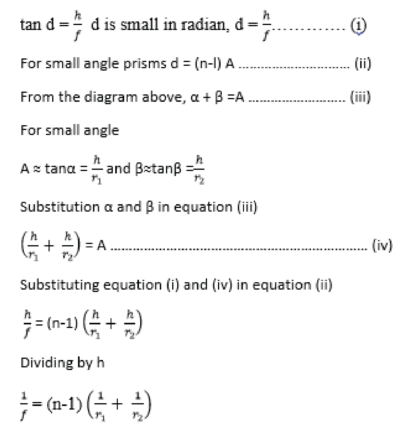
(d) Describe an experiment to determine the focal length of a thin converging lens mounted inside a short cylindrical tube. (05marks)

(e) A compound microscope consists of two thin lenses, an objective of focal length 1.0cm and eye piece of focal length 5.0cm. The objective forms an image of an object placed in front of it at a point 16.0cm away. If the final image is formed at the near point of the eye, calculate the

(i) Separation of the lenses (03marks)

(ii) magnifying power of the instrument (03marks)

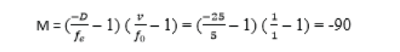
7. (a) (i) Describe how the focal length of a convex mirror can be measured using a convex lens of known focal length. (04marks)

Determination of the focal length of a convex mirror can be measured using a convex lens of known focal length.

 

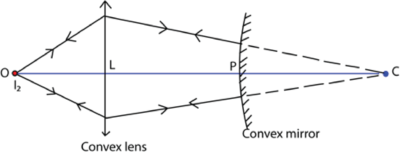
• The apparatus is arranged as shown above
• An object, O is placed in front of a convex lens L and its image formed at C
• The distance LC is measured and recorded.
• The convex mirror whose focal length, f, is required is placed is placed between L and C with its reflecting surface facing the lens.
• The lens is then moved along the axis, OC until a converging beam incident normally on the mirror forms its image at O
• Distance LP is measured

          PC = LC – LP thus, f can be determined from 

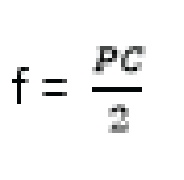
(ii) The plane mirror, P, in the figure below is adjusted to a position20cm from optical pin, the image of the pin in P coincides with its image in M.

Calculate the focal length of the convex mirror. (04marks)

(b) A pin is clamped horizontally above a concave mirror with its tip along the principal axis. When the pin is adjusted, it coincides with its image at a distance R from the mirror. When a small liquid of refractive index, n, is put on the mirror, the pin again coincides with its image at a distance R’ from the mirror. Show that the refractive index, n, is given by

  (04marks)

(c)(i) Explain the term eye-ring as applied to the telescope. (02marks)

The eye-ring is the image of objective lens in eyepiece

(ii) Draw a ray diagram to show the formation of final image in Galilean telescope in normal adjustment. (03marks)

(iii) Explain two advantages and one disadvantage of the telescope in (c)(ii) above. (03marks)

Advantages of a Galilean telescope

It forms a final erect image.

It is shorter than the terrestrial and astronomical telescopes because the separation of lenses is

f₀-f_e.

Disadvantages of a Galilean telescope

It has a small field of view.

It has a virtual eye ring not accessible to the observer

8. (a)(i) When does light pass through a prism symmetrically? (01marks)

Light is said to pass through a prism symmetrically when the angle of incidence is equal to the angle of emergence or when minimum deviation occurs.

(ii) Find the angle of incidence, i, on an equilateral prism of refractive index 1.5 placed in air, when light passes through it symmetrically. (03marks)

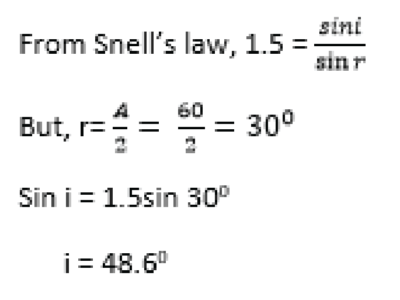
(iii) Describe what happens to the deviation of light passing through a prism in (a) (ii) when the angle of incidence is increased from a value less than i to a value greater than i. (02marks)

Angle of deviation increases when either angle i is increased or decreased.

(b) Describe how the refractive angle of a prism can be determined using optical pins. (05marks)

Using optical pins

A white paper is stuck to the soft board using top-headed pins. Two parallel lines AB and DC are drawn on the paper and the prism is placed with its apex as shown.

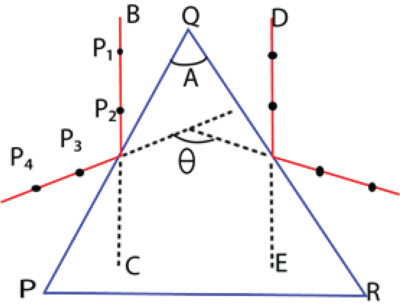
• Two optical pins P1and P2 are placed along AB and pins P3 and P4 are placed such that they appear to be in line with the images of P₁and P2 as seen by reflection from face P
• The procedure is repeated for face The prism is removed and angle θ is measured..

           The required refracting angle A   = θ/2

 

(c) (i) Draw a sketch ray diagram showing formation of the image of a finite size real object by a concave lens. (02marks)

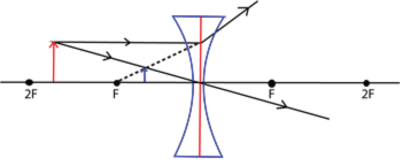
(ii) A concave lens of focal length 15.0cm is arranged coaxially with a concave mirror of focal length 10.0cm, a distance of 4.0cm apart. An object is placed 20.0cm in from of the lens on the side remote from the mirror. Find the distance of the final image from the lens. (04marks)

(d) With the aid of a sketch ray diagram explain spherical aberration in a concave lens, and state how it is minimized. (03marks)

When a wide beam of light falls on the lens, marginal rays are refracted and appear to come from a point different from that of the central rays.

The image formed is thus blurred (distorted). This is called spherical aberration. It is minimized by using an opaque disc with central hole to allow only central rays to pass through

 

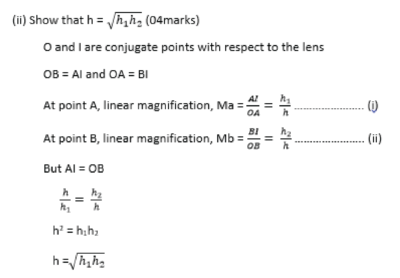
9. (a) Explain what is meant by conjugate points. (02marks)

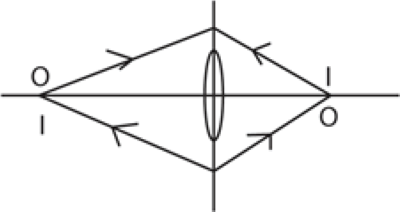
These are two points, O and I. each on the opposite side of a convex lens such that an object and its image at these points are interchangeable.

(b) A converging lens forms an image of height, h₁ on a screen of an object O of height, h. When the lens is displaced towards the screen, an image of height, h₂ is formed on the screen.

(i) Sketch a ray diagram to show the formation of the images on the screen. (02marks)

 

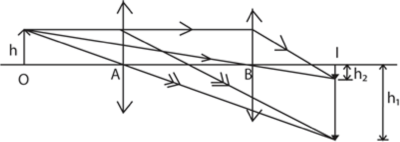
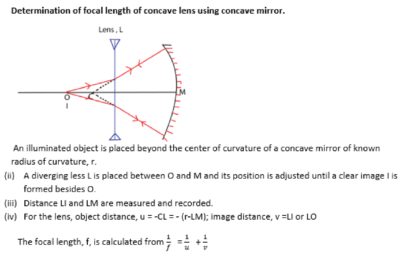
(c) Describe an experiment to determine focal length of a diverging lens using a concave mirror of known focal length.

(d) The objective of astronomical telescope in normal adjustment has a diameter of 150mm and focal length of 3.0m. The eyepiece has focal length of 25.0mm. Calculate

(c) Give one advantage of placing the eye at the eye-ring (01mark)

A clearest image is observed.

 

10. (a) show that for a ray of light passing through layers of transparent media separated by parallel boundaries, nsini = a

where a is a constant and n is the refractive index of the medium containing angle i. (04marks)

Consider a ray of light moving from air through a series of media 1, 2 and then finally emerge into air as shown.

(b) (i) What is meant by critical angle? (01mark)

Critical angle is the angle of incidence in a denser medium for which the angle of refraction in a less dense medium is 90⁰.

(ii) Describe an experiment to determine the critical angle for a water-air boundary. (05marks)

(c) The figure below shows an isosceles prism ABC of refractive index 1.51, dipped in a liquid with refractive edge downwards. A ray of light incident on the prism at an angle i = 34.6⁰ emerges perpendicularly through the base.

(i) Calculate the refractive index of the liquid (04marks)

Solution

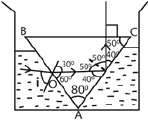
The angles are represented on the diagram above, r = 30⁰

From nsin i = constant

nsin34.6 = 1.51sin30

n = 1.33

 

(d) Explain how an optical cable transmits light. (03marks)

An optical fiber is made of a transparent material coated with another less optically dense material. Light entering the pipe strikes the boundary of the media at an angle of incidence greater than the critical angle. Total internal reflection thus occurs. This takes place repeatedly in the pipe until the light beam emerges from the pipe.

 

(e) An optical pin held above a concave mirror containing water of refractive index 1.33, coincides with its image at a distance of 12cm above the mirror. When the water is replaced by a little quantity of a certain liquid, the point of coincidence of the object and the image becomes 13.3cm. Calculate the refractive index of the liquid. (03marks)

 

11. (a) (i) State the laws of reflection of light. (02marks)

• The incident ray, the reflected ray and the normal at the point of incidence all lie in the same plane
• The angle of incidence is equal to the angle of reflection

(ii) A ray of light is incident on a plane mirror. The mirror is then turned through an angle α keeping the direction of the incident ray constant. If a reflected ray turned through angle β, find the relationship between α and β.

Let XY be the initial position of the mirror with ray AO making a glancing angle g. By keeping the direction of the incident ray fixed, the mirror is rotated through an angle α to a new position X’Y’ as shown.

 

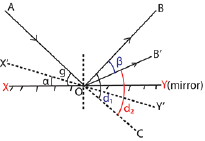
Case 1 (mirror in position XY)

Glancing angle = g

Deviation d₁ = 2g……… (i)

Case 2(mirror in position X’Y’)

Glancing angle = (g – α)

Deviation d₂ = 2(g – α) …..(ii)

β = d₁ –d₂

   = 2g – 2(g – α)

   = 2g-2g + 2α

  = 2α

(b) Describe how a sextant is used to determine the angle of elevation of a star. (05marks)

Setup

• A sextant consists of a fully silvered mirror M1 which can be rotated about a horizontal axis and a fixed half silvered mirror B.
• Mirror M1 is adjusted to become parallel to B by rotating it until the image of the horizon, H’ is seen directly through the unsilvered part of mirror B by successive reflection in mirror M₁ and B respectively
• The mirror M₁ is rotated to position M₂ such that the image of the horizon H, and the sun coincides at H’
• The angle of rotation is measured from the scale on the instrument. The elevation of the sun is 2θ.

(c) Describe an experiment to determine the refractive index of a small quantity of a liquid using a concave mirror. (05marks)

An experiment to determine refractive index of a liquid using a concave mirror

                                   

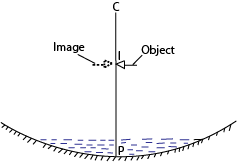
• A clamped pin with its tip along the principal axis above the concave mirror is coincided with its image at C and distance PC is measured
• A small quantity of the liquid under test is poured into a concave mirror and a new point I at which the object pin coincides with its image is obtained.
• Distance IP is measured.
• The required refractive index of a liquid,

                                                   

 

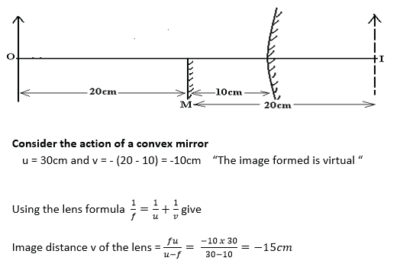
(d) A plane mirror is placed 10cmin front of a convex mirror so that it covers about half of the convex mirror surface. A pin placed 20cm in front of the plane mirror gives an image which coincides with that of the pin in the convex mirror. Find the focal length of the convex mirror. (04marks)

12. (a) Define angular magnification of an optical instrument. (01marks)

This is the ratio of the angle subtended at the eye by the image when using an instrument to the angle subtended at unaided eye by the object.

(b) (i) Describe with the aid of a ray diagram, the operation of telescope made of a converging lens  and a diverging lens when used in normal adjustment. (05mrks)

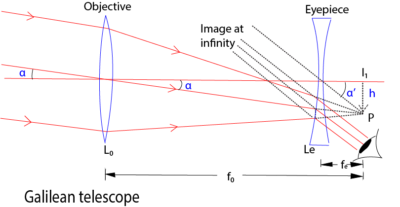
Light from distant object incident on objective lens is refracted to form real inverted image at its principal focus f₀. In normal adjustment, the principal focuses of both lenses coincide. The eye piece therefore forms the final image of the object at infinity.

(ii) State two limitations of this type of telescope. (01marks)

• it has virtual eye-ring
• It has a small field of view
• It produces less clear image

(c) The diagram in the figure below shows a path followed by a ray of monochromatic light through a right angled prism of refractive index 1.52. The light emerges in air at an angle of 47.4⁰.

Find the refractive index of the liquid. (06marks)

 

Solution

Note: A ray on the boundary is grazing ray.

Let the refractive index of the liquid and air be and c is the refractive index of glass.

From

n_g sini = na sin 47.6⁰

1.52 sin r = 1sin 47.6⁰

             r  = 29⁰

       r + c = 90⁰

            c = 61⁰

n_Lsin 90⁰ = ng sin c

       n_L = 1.52 sin 61⁰ = 1.33

 

(d) Explain the following as applied to lenses.

(i) chromatic aberration (02marks)

It is a failure of a lens to focus all colors to the same point due to different refractive indices for the different color wavelength.

(ii) spherical aberration (02marks)

     It is the failure of the lens to focus all rays through the lens to the same point leading blurred image

(e) Explain how chromatic and spherical aberration are minimized in a reflecting telescope (03marks)

 

Correction of chromatic aberration

• Use lenses made of low-dispersion glasses, especially those containing fluorite
• By using combination of lenses of opposite nature (convex & concave) or chromatic doublet such that the dispersion produced by one lens is reversed by another.
• Placing the eye close to the lens such that images due to different colours subtend the same angle at the eye.

Means of reduction of spherical aberration

• Using lenses with an aspheric surface
• Using lens of small aperture.
• Using a stopper such that only light incident on the middle of the lens pass, but this method reduces the brightness of the image since it reduces the amount of light energy passing through the lens.

 

 

13. (a) Distinguish between a real and virtual image (02marks)

A real image is formed by actual intersection of rays and can be formed on a screen while a virtual image is formed by apparent intersection of rays and cannot be formed on a screen.

(b) Derive an expression relating the focal length, f, of a convex mirror to the object distance, u and image distance, v.

Consider the incidence of ray OX on to a convex mirror from a point object O placed along the principle axis and then suddenly reflected in the direction XK making an angle q with the normal XN.

 

(c) A convex mirror forms an image half the size of the object. The object is then moved towards the mirror until the image is three quarter that of the object. If the image is moved by a distance of 0.6cm, calculate

(i) focal length of the mirror. (03marks)

(d) (i) What is critical angle? (01mark)

  Critical angle is the angle of incidence in the dense medium for which the angle of refraction                in the less dense medium is 90⁰.

(ii) Explain how mirage is formed (04marks)

On a hot day, layer on air near the ground are hotter and lens dense than layers above. This leads to total internal reflection of rays of light from the sky. And mirage is the image of the sky to the eye by total internal reflection

(e) State four applications of total internal reflection (02marks)

• in radio broadcasting
• determination of refractive index if material
• in optical fiber transmission
• in refracting prisms in binoculars and periscopes

14. (a) State laws of refraction. (02marks)

• The incident ray, the refracted ray and the normal at the point of incidence all lie in the same plane
• The ratio of the sine of angle incidence to the angle of refraction is constant for a given pair of the media.

(b) (i) The deviation, d, by small angle prism of refractive angle A and refractive index, n, is given by

d = A(n-1)

Use this expression to show that the focal length, f, of a thin converging lens of refractive index, n, is given by

                     ,

where r₁ and r₂ are radii of curvature of the lens surfaces (05marks)

Solution

Consider a ray close and parallel to the principal axis, incident at height, h.

(ii) The figure below is a glass convex lens in air with surfaces A and B having radii of curvature 10cm and 15cm respectively.

(c) (i) With the aid of a ray diagram, describe the structure and action of a Galilean telescope in normal adjustment. (05marks)

Light from distant object incident on objective lens is refracted to form real inverted image at its principal focus f₀. In normal adjustment, the principal focuses of both lenses coincide. The eye piece therefore forms the final image of the object at infinity.

 

(ii) Derive an expression for angular magnification of the telescope in (c)(i). (03marks)

(d) Explain the disadvantage of a Galilean telescope over refracting type. (02marks)

• Eye-ring is virtual
• Image less bright and less clear

15. (a) (i) State the laws of refraction of light (02marks)

• The incident ray, the refracted ray and the normal at the point of incidence all lie in the same plane
• The ratio of the sine of angle incidence to the angle of refraction is constant for a given pair of the media.

(ii) State the conditions for total internal reflection to occur. (02marks)

• Angle of incidence must be greater than the critical angle.
• Light must be moving from a denser to less dense medium

(b) (i) Describe an experiment to determine refractive index of a liquid using air cell. (06marks)

(ii) Explain the difficulty encountered in the experiment described in (b)(i) if white light is used (02marks)

White light does not give sharp extinction of light due to dispersion.

 

(c) A cube of glass of side 3cm and refractive index 1.5 is placed on a thin film of liquid as shown in the figure below

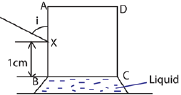
A ray of light in the vertical plane strikes AB of the glass cube at an angle i = 410. After refraction at X, the ray is reflected at the critical angle for glass-liquid interface.

(i) Calculate the critical angle for glass –liquid interface. (03marks)

Solution

At point P,

From nsin i = constant

sin (90 – 41) = 1.5sin r

                r = 30.2⁰

At point Q

r + c =90⁰

c = 90⁰ – 30.2⁰ = 59.8⁰

 

(ii) Find the position from B where the ray strikes the glass-liquid interface

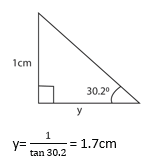
(d) Explain why the rays from the sun can still be seen shortly after sunset (03marks)

As the sun sets, layers of air near the earth gets cooler and therefore optically denser than layers of the air above. This causes total internal refraction of light rays from the sun towards the earth.

 

16. (a) with the aid of ray diagram, explain the following as applied to lenses

(i) conjugate points. (02marks)

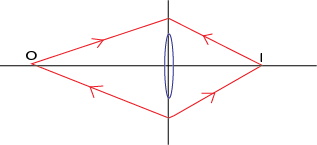
These are two points; O and I, on the opposite side of a convex lens such that an object and its image at these points are interchangeable.

(ii) spherical aberration (02marks)

Spherical aberration

           

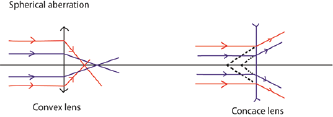
Spherical aberration occurs when the lens fails to focus all rays falling on it to the same point. Thus images formed by the lens at large apertures are therefore unsharp or blurred at the edges.

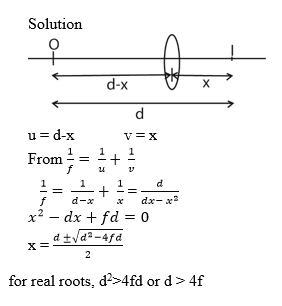
(b) An object, O, placed in front of a converging lens forms a real image, I, on the screen. The distance between the object and its real image is d. while that of the image from the lens is x.

Derive the expression for the least possible distance between the object and its real image (05marks)

(c) Give the properties of lenses in achromatic combination. (03marks)-

• One lens should be concave and the other convex
• The lenses should be of different materials
• The dispersion caused by the concave lens should be completely cancelled by the convex lens. 
• The radii of curvature of concave and convex lenses should be numerically equal

(d) A compound microscope consists of two converging lenses of focal lengths 1.0cm and 5.0cm respectively. An object is placed 1.1cm from objective and the microscope is adjusted so that the final image formed 30cm from the eye- piece. Calculate

(i) the separation of the lenses (03marks)

(ii) the magnifying power of the lenses. (03marks)

(e) State two differences between a compound microscope and an astronomical telescope. (02marks)

 

Compound microscope	Astronomical telescope
–  view near objects	– views distant objects
– objective lens has smaller focal length	– objective has longer focal length
– in normal adjustment, final image is at near point	– in normal adjustment final image is at infinity
– has greater resolving power	-has smaller resolving power

 

17. (a) Define the following terms as applied to a concave lens:

(i) principal focus’ (01mark)

it is appoint on principal axis where rays of light originally parallel and close to principal axis appear to diverge from after reflection by the lens.

(ii) radii of curvature (01mark)

are radii of the sphere of which the lens surfaces form part

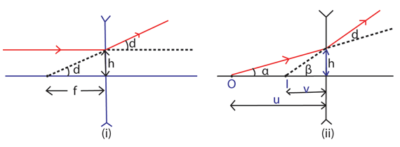
(b) A point object is placed at a distance u in front of a diverging lens of focal length, f, to form an image at a distance, v, from the lens.

Derive an expression that relates u. v. and f. (04marks)

Consider in each case a ray incident on the same lens at a small height h above the principal axis as shown:

 

(c) Describe an experiment to determine the focal length of a concave lens using a plane mirror, converging lens and illuminated object. (04marks

Setup

• The apparatus is shown above
• The wire gauze is illuminate with a bulb and the position of lens L₁ is adjusted until a sharp image of the wire gauze is formed on the object screen, S.
• The distance SL₁ is measured and recorded as f₁.
• The test lens L₂ is now cemented of L₁ and again placed between O and M.
• The position of the combined lens is adjusted until a sharp image of the wire gauze is formed on S.
• The distance SL₂ is measured as f₂.
• Focal length of the test lens, f, is then calculated from,

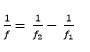
(d) What is meant by a:

(i) visual angle (01mark)

Visual angle is the angle subtended by an object or image at the eye.

(ii) near point (01mark)

The near is the point at which the eye is able to view the object clearly with the greatest details.

(e) A person with a normal near point distance of 25cm wears spectacles with a diverging lens of focal length 200cm in order to correct the far point distance to infinity. Calculate the near point when viewing using the spectacles. (03marks)

(f) (i) Draw a ray diagram to show the formation of an image of a distant object in terrestrial telescope in normal adjustment. (03marks)

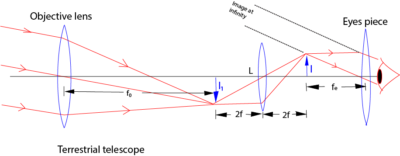
(ii) State two disadvantages of terrestrial telescope.(02marks)

• Long and bulky
• Extra diffraction occurs in erecting lens reducing the clarity of the final image

 

18. (a) What is meant by the term:

(i) refraction (01mark)

Refraction is the change in direction of propagation of light as it travels from one medium to another.

(ii) absolute refractive index? (01mark)

Absolute refractive index of a medium is the ratio of speed of light is vacuum to the speed of light in the medium.

(b) Describe an experiment to determine the refractive index of a liquid using a travelling microscope. (04marks)

Measurement of refractive index of a liquid using travelling microscope or by apparent depth method.

A vertically traveling microscope having a graduated scale T besides it is focused on sand particles placed at O on bottom of the container. The scale reading a on T

is noted.

A liquid whose refractive index is required is filled in a container and the microscope is raised until the particles are refocused at I. The scale reading b is again noted.

Finally the traveling microscope is focused on the liquid surface giving a scale reading c.

 

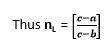
(c) The figure below shows monochromatic light X incident towards A on vertical screen.

 

 

When the semicircular glass block is placed across the path of light with its flat face parallel to the screen, a bright spot is formed at A. When the glass block is rotated about a horizontal axis through O, the bright sport moves from A to B and then just disappears. At B, distance 1.68cm from A.

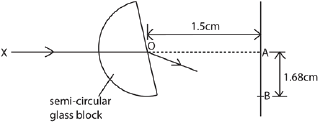
(i) Find the refractive index of the material of the glass block. (04marks)

(ii) Explain whether AB would be longer or shorter if the block of glass of higher refractive index was used.

When the refractive index is high the ray is refracted more, AB becomes longer.

(d) (i) A ray of monochromatic light is incident at a small angle of incidence on a small-angle prism in air. Obtain the expression, d= (n-1)A, for the deviation, d, of light by the prism, where A is the refracting angle of the prism and n, is the refractive index. (04marks)

Solution

The small refracting angle of this prism causes the angle i₁, r₁, r₂ and i₂ to be small such that sin i₁ ≈ i₁, sin r₁ ≈ r₁ sin r₂ ≈r₂ and sin i₂ ≈ i₂

From the diagram, d = d1 + d₂

But   d₁ = i₁ – r₁ and d₂ = i₂ – r₂

=> d =  (i₁ – r₁)  + (i₂ – r₂)

On simplifying d = i₁ + i₂ – (r₁ + r₂)

but r1 + r₂   = A

=>  d  = i₁  + i₂ – A ……………………………………………… ……(a) 

At M Snell’s law becomes.

na sin i₁ = n sin r₁

For small angles this gives i₁   = nr₁ ……………………………………(b)

Similarly at N Snell’s law becomes    i₂ = nr₂ …………………………(c)

Substituting equation (b) and (c) in (a) gives

d = nr₁ + nr₂ – A

d = n (r₁ + r₂) –A

but   r₁ + r₂ = A

=> d = nA  – A

 d = (n – 1)A

 

(ii) Calculate the minimum deviation produced by a 60⁰ prism if the refractive index of the glass is 1.50. (03marks)

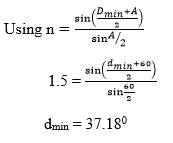
• State any two applications of total internal reflection. (01marks)
• Transmission of light by optical fiber
• Reflecting prisms in binoculars, periscopes

 

19. (a) (i) Define principal focus of a concave lens. (01marks)

Principal focus of a concave lens is the point on the principal axis where rays of light parallel and close to principal axis appear to diverge from after refraction through the lens.

(ii) Draw a ray diagram to show formation of an image of finite object in a concave lens (02marks)

(iii) Describe the image formed in (a)(ii) (01mark)

– it is virtual and diminished.

 

(iii) Explain what would happen if the lens was replaced with a similar one but of much smaller focal length. (03marks)

If the lens was replaced with one of a smaller focal length, the final image would have been smaller. This is because the power of a lens = ; therefore a lens of smaller focal length has a larger magnifying power.

(b) Explain how spherical aberration is minimized in a photographic camera.(03marks)

Spherical aberration in a photographic camera is minimized

• by the diaphragm. The diaphragm only allows central beam which forms a sharp image.
• using composite lens

 

20. (a) Define refractive index. (01mark)

Refractive index of a medium is the ratio of the speed of light in vacuum to the speed of lght in a medium.

(b) (i) Describe with the aid of a diagram, how the refractive index of a liquid can be determined using air cell. (05marks)

(ii) Derive the expression used to obtain the refractive index of the liquid in (b)(i) (03marks)

Setup

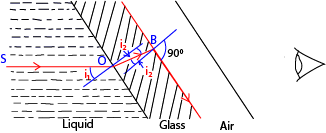
Ray SO is refracted along OB in glass. However, at B total internal just begins. Suppose i₁ is the angle of incidence in the liquid, i₂ is the angle of incidence in the glass  while n and ng are the corresponding refractive indices, Then applying the relation n sin i = a constant gives

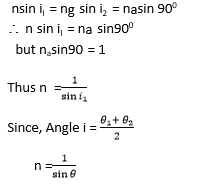
(c) A prism of refractive angle 60⁰ has refractive indices 1.515 and 1.529 for red and violet respectively. When white light is incident on one face of the prism, red light undergoes minimum deviation. Calculate the angle of

(i) incidence for white light (04marks)

(ii) emergence for violet light(03marks)

(d) Describe the adjustment that have to be made before a spectrometer can be used. (04 marks)

• The collimator is adjusted to produce parallel rays of light
• The turn table is levelled
• The telescope is adjusted to receive light from the collimator on its cross wire.

 

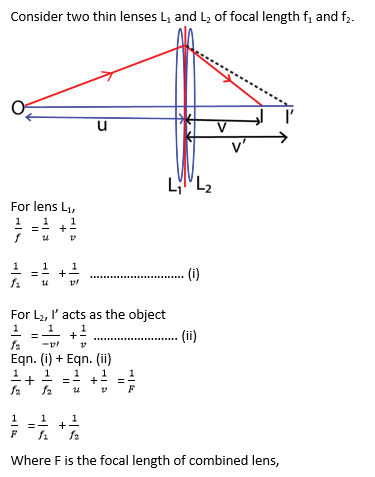
21. (a) (i) Show that the effective focal length, f, of two thin lenses in contact is given by

where f₁ and f₂ are the foal lengths of the individual lenses. (04marks)

 

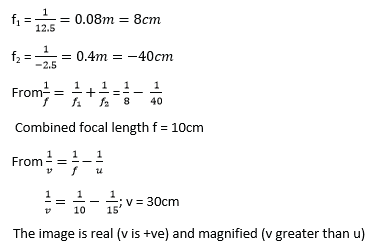
(ii) A compound lens consists of two lenses in contact having powers of +12.5D and -2.5D. Find the position and nature of the image of an object placed15.0cm from the compound lens. (03marks)

(b)(i) Define refractive index (01marks)

Refractive index is the ratio of the speed of light in vacuum to the speed of light in a medium

Or

The ration of sine of angle of incidence of a ray moving from vacuum to the sine of angle of refraction in a medium

(ii) An equi-convex lens is placed on a horizontal plane mirror and a pin held vertically above the lens is found to coincide with its image when positioned 20.0cm above the lens. When a few drops of liquid is placed between the lens and the mirror, the pin had to be raised 10.0cm to obtain coincidence with the image. If the refractive index of the lens is 1.5, find the refractive index of the liquid. (05marks)

(c) (i) What is meant by magnifying power of optical instrument? (01mark)

Magnifying power of optical instrument is the ratio of the angle subtended by the final image at the eye when using optical instrument to the angle subtended by the object at the naked eye.

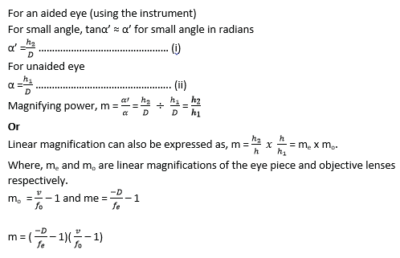
(ii) Derive an expression for magnifying power of a compound microscope in normal adjustment. (05marks)

 

(ii) Why should the objective and eye piece of a compound microscope have short focal length? (01 marks)

To achieve a bigger magnification

Additional questions download the pdf:                          Geometrical optics revision questions (A-level) with answers

Share with a friend

Thank you so much

Compiled by Dr. Bbosa Science