Physical quantities

Physical quantities are divided into two groups

(a) Fundamental quantities

These are physical quantities that cannot be expressed in the form of other quantities using any mathematical equations. They include

Quantity	S.I unit	Symbol of S.I unit
Mass	kilogram	kg
Time	second	s
Length	metres	m
Temperature	Kelvin	K
Current	Ampere	A

(b) Dimensional/derived quantities

These are physical quantities which can be expressed in terms of fundamental quantities. For example velocity, work, volume, density

Dimensions of physical quantities

This is a way in which derived quantities can be expressed in form of fundamental quantities. i.e.

Mass- M

Length- L

Time –T

The square bracket, [   ] is used to show dimensions

For example

(i) [Area] = length x length or length x width

                                  L x L = L²

(ii) Volume = length x width x height

  =  L x L x L = L³

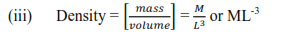
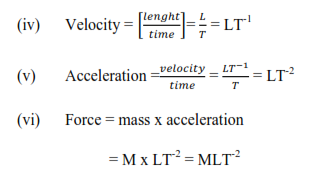
 Application of dimensions

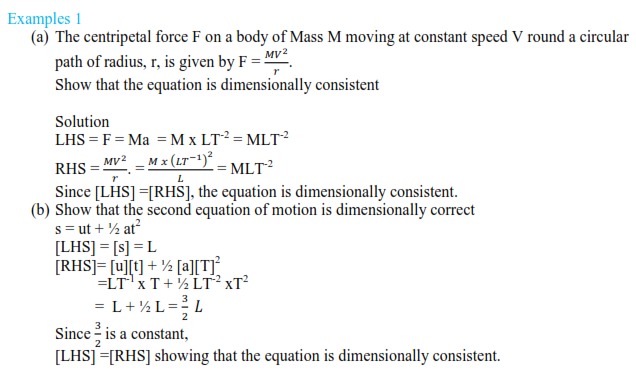
(i) To check the validity of equation (equations that are not dimensionally consistent are obviously wrong expression and should be discorded.

(ii) To derive equations: for correct equation, the units of the left hand side must be similar to the units of the right hand side.

All right equations must be dimensionally consistent but not all dimensionally consistent equations are correct.

Derivation of equation

The method of dimensions can be used to derive equations.

Examples 2

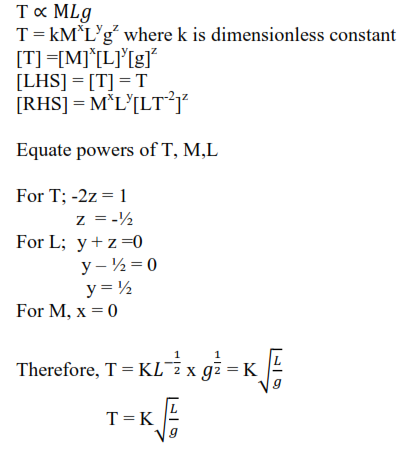
The period (T) of a pendulum bob depends on the length of the pendulum (L), mass of the pendulum ball,  M, and acceleration due to gravity, g. Determine  an expression for the period of a simple pendulum T in terms of the quantities mentioned.

Solution

Exercise

1. The sphere of radius, α, moving through a liquid of density, ρ, and velocity, v, experiences a retarding force given by F = kα^xρ^yv^z, where K is a non dimensional constant. Use dimensions to find the values of x, y and z. [Ans, y =1, z =2 , y = 2]

2. Use dimensional analysis to show how the process of velocity transverse process  vibration of a stretched string depends on its length, L, mass, m, and the tension F of the string V = KL^xM^yF^z, where k is a non-dimensional constant. Find the values of x, y, z.

[Ans, x = ½ , y = – ½ , z = 1/2]

3. A cylindrical vessel of cross section area, A, contains air of volume, V, atmospheric pressure, ρ, trapped by frictionless down and released.

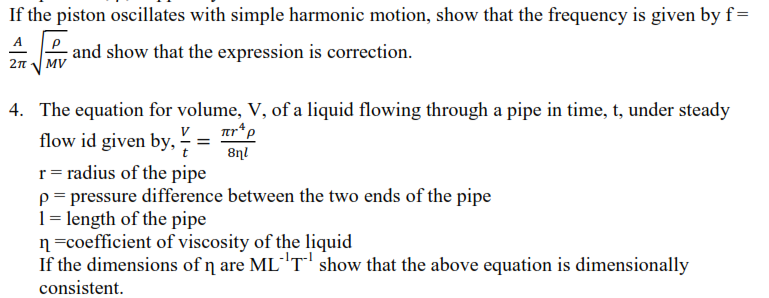
5.For streamline flow of a non-viscous incompressible fluid , the pressure, ρ, at a point is related to height, h. and velocity, V, by the equation

(p-a)=pg(h-b) + ½ p(v² –d)

Where a, b, and d are constant and p is the density of fluid   and g is the acceleration due to gravity. Given that the equation is dimensionally consistent. Find the dimensions of a, b, and d.

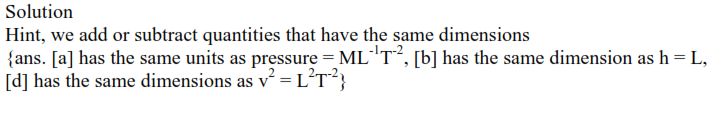
Sponsored by The Science Foundation College + 256 753 802709

Dr. Bbosa Science